Hostname: page-component-78c5997874-8bhkd Total loading time: 0 Render date: 2024-11-05T09:29:11.784Z Has data issue: false hasContentIssue false
  • English
  • Français

Discrete Space-time and Lorentz Transformations

Published online by Cambridge University Press:  20 November 2018

Gerd Jensen  and
Christian Pommerenke
Gerd Jensen
Affiliation:
Sensburger Allee zz a, D–14055 Berlin e-mail: [email protected]
Christian Pommerenke
Affiliation:
Institut für Mathematik, Technische Universität, D–10623 Berlin e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Alfred Schild established conditions where Lorentz transformations map world-vectors 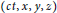$\left( ct,\,x,\,y,\,z \right)$ with integer coordinates onto vectors of the same kind. The problem was dealt with in the context of tensor and spinor calculus. Due to Schild’s number-theoretic arguments, the subject is also interesting when isolated from its physical background.

Schild’s paper is not easy to understand. Therefore, we first present a streamlined version of his proof which is based on the use of null vectors. Then we present a purely algebraic proof that is somewhat shorter. Both proofs rely on the properties of Gaussian integers

Keywords

22E4320H9983A05Lorentz transformationinteger latticeGaussian integers
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 59 , Issue 1 , 01 March 2016 , pp. 123 - 135
Copyright
Copyright © Canadian Mathematical Society 2016

References

[1] Baker, A., Matrix groups. Springer, 2002.Google Scholar
[2] Hardy, G. H. and Wright, E. M., An introduction to the theory of numbers. Fifth éd., The Clarendon Press, Oxford University Press, New York, 1979.Google Scholar
[3] Horn, R. A. and Johnson, C. R., Topics in matrix analysis. Cambridge University Press, Cambridge, 1991.Google Scholar
[4] Lorente, M. and Kramer, P.. Representations of the discrete inhomogeneous Lorentz group and Dirac wave equation on the lattice. J. Phys. A 32(1999), no. 12, 2481–2497. http://dx.doi.Org/10.1088/0305-4470/32/12/01 9 Google Scholar
[5] Louck, J. D., A new parametrization and all integral realizations of the Lorentz group. J. Math. Phys. 43(2002), 5108–5134. http://dx.doi.Org/10.1 063/1.1 505124 Google Scholar
[6] Penrose, R. and Rindler, W.. Spinors and space-time. Vol. I, Cambridge Monographs on Mathematical Physics Cambridge University Press, Cambridge, 1984. http://dx.doi.Org/10.1017/CBO9780511 564048 Google Scholar
[7] Schild, A., Discrete space-time and integral Lorentz transformations. Canad. J. Math. 1(1949), 29–47. http://dx.doi.Org/10.4153/CJM-1949-003-4 Google Scholar