No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
Let A be an m×n (0, l )-matrix. Let C1, C2, …, Cn denote its columns. A sequence of distinct columns 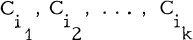 is said to form a chain if the inner product of
is said to form a chain if the inner product of 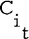 and
and 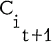 (for 1 ≤ t ≤ k-l) is at least one. k-1 is called the length of the chain and this chain is said to connect
(for 1 ≤ t ≤ k-l) is at least one. k-1 is called the length of the chain and this chain is said to connect 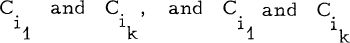 are said to be connected. As can be easily seen, connectedness is an equivalence relation on the set of columns. A matrix is called connected if all its columns belong to the same equivalence class. If Ci and Cj belong to the same equivalence class, then s(Ci, Cj) will denote the length of the shortest chain between Ci and Cj We define the distance between any two columns Ci and Cj to be denoted by d(Ci, Cj), in the following manner.
are said to be connected. As can be easily seen, connectedness is an equivalence relation on the set of columns. A matrix is called connected if all its columns belong to the same equivalence class. If Ci and Cj belong to the same equivalence class, then s(Ci, Cj) will denote the length of the shortest chain between Ci and Cj We define the distance between any two columns Ci and Cj to be denoted by d(Ci, Cj), in the following manner.
This work was done while the author was at the University of Alberta, Edmonton.