Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-23T19:38:06.476Z Has data issue: false hasContentIssue false
  • English
  • Français

φ-Dialgebras and a Class of Matrix “Coquecigrues”

Published online by Cambridge University Press:  20 November 2018

Fausto Ongay
Fausto Ongay*
Affiliation:
CIMAT, Guanajuato, Gto., 36240 Mexico e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Starting with the Leibniz algebra defined by a 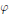$\varphi$-dialgebra, we construct examples of “coquecigrues,” in the sense of Loday, that is to say, manifolds whose tangent structure at a distinguished point coincides with that of the Leibniz algebra. We discuss some possible implications and generalizations of this construction.

Keywords

14M30Leibniz algebrasdialgebras
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 50 , Issue 1 , 01 March 2007 , pp. 126 - 137
Copyright
Copyright © Canadian Mathematical Society 2007

References

[F] Felipe, R., Generalized Loday algebras and digroups. Submitted to J. Pure Appl. Algebra, 2003Google Scholar
[FLOV] Felipe, R., López-Reyes, N., Ongay, F., and Velásquez, R., Yang-Baxter equations on matrix dialgebras with a bar unit. Linear Algebra Appl. 403(2005), 31–44.Google Scholar
[K] Kinyon, M. K., Leibniz algebras, lie racks, and digroups. arXiv.math.RA/0403509.Google Scholar
[KW] Kinyon, M. K. and Weinstein, A., Leibniz algebras, courant algebroids, and multiplication on reductive homogeneous spaces. Amer. J. Math. 123(2001), no. 3, 525–550.Google Scholar
[KN] Kobayashi, S. and Nomizu, K., Foundations of Differential Geometry. Vol. 1, Wiley, New York, 1963.Google Scholar
[L] Liu, K., A class of group-like objects. arXiv.math.RA/0311396.Google Scholar
[L1] Loday, J. L., Une version non commutative des algèbres de Lie: les algèbres de Leibniz. Enseign. Math. 39(1993), no. 3–4, 269–293.Google Scholar
[L2] Loday, J. L., Dialgebras. In: Dialgebras and Related Operads, Lecture Notes in Math. 1763 Springer-Verlag, Berlin, 2001, pp. 7–66.Google Scholar