Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-25T15:16:08.618Z Has data issue: false hasContentIssue false
  • English
  • Français

Deciding the Existence of Minority Terms

Part of: Theory of computing General logic Algebraic structures

Published online by Cambridge University Press:  24 October 2019

Alexandr Kazda ,
Jakub Opršal ,
Matt Valeriote  and
Dmitriy Zhuk
Alexandr Kazda
Affiliation:
Charles University, Prague, Czech Republic Email: [email protected]
Jakub Opršal
Affiliation:
University of Durham, Durham, UK Email: [email protected]
Matt Valeriote
Affiliation:
McMaster University, Hamilton, Ontario, Canada Email: [email protected]
Dmitriy Zhuk
Affiliation:
Charles University, Prague, Czech Republic Lomonosov Moscow State University, Moscow, Russia Email: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper investigates the computational complexity of deciding if a given finite idempotent algebra has a ternary term operation $m$ that satisfies the minority equations 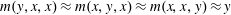$m(y,x,x)\approx m(x,y,x)\approx m(x,x,y)\approx y$. We show that a common polynomial-time approach to testing for this type of condition will not work in this case and that this decision problem lies in the class NP.

Keywords

Maltsev conditionminority termcomputational complexity

MSC classification

Primary: 68Q25: Analysis of algorithms and problem complexity
Secondary: 03B05: Classical propositional logic 08A40: Operations, polynomials, primal algebras
Type
Article
Information
Canadian Mathematical Bulletin , Volume 63 , Issue 3 , September 2020 , pp. 577 - 591
Check for updates
Copyright
© Canadian Mathematical Society 2019

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Author A. K. was supported by Charles University grants PRIMUS/SCI/12 and UNCE/SCI/022; J. O. was supported by the European Research Council (Grant Agreement no. 681988, CSP-Infinity) and the UK EPSRC (Grant EP/R034516/1); M. V. was supported by the Natural Sciences and Engineering Council of Canada; D. Z. was supported by the Russian Foundation for Basic Research (grant 19-01-00200).

References

Bergman, C., Universal algebra: Fundamentals and selected topics. Pure and Applied Mathematics (Boca Raton), 301, CRC Press, Boca Raton, FL, 2012.Google Scholar
Bulatov, A., Mayr, P., and Szendrei, Á., The subpower membership problem for finite algebras with cube terms. Log. Methods Comput. Sci. 15(2019), no. 1, Paper No. 11.Google Scholar
Burris, S. and Sankappanavar, H. P., A course in universal algebra. Graduate Texts in Mathematics, 78, Springer-Verlag, New York-Berlin, 1981.CrossRefGoogle Scholar
Dalmau, V., Generalized majority-minority operations are tractable. Log. Methods in Comput. Sci. 2(2006), no. 4, 4:1. https://doi.org/10.2168/LMCS-2(4:1)2006.CrossRefGoogle Scholar
Freese, R. and Valeriote, M. A., On the complexity of some Maltsev conditions. Internat. J. Algebra Comput. 19(2009), 41–77. https://doi.org/10.1142/S0218196709004956CrossRefGoogle Scholar
García, O. C. and Taylor, W., The lattice of interpretability types of varieties. Mem. Amer. Math. Soc. 50(1984), no. 305. https://doi.org/10.1090/memo/0305.Google Scholar
Horowitz, J., Computational complexity of various Mal’cev conditions. Internat. J. Algebra Comput. 23(2013), 1521–1531. https://doi.org/10.1142/S0218196713500343CrossRefGoogle Scholar
Kazda, A. and Valeriote, M., Deciding some Maltsev conditions in finite idempotent algebras. J. Symbolic Logic., to appear.Google Scholar
Kearnes, K. A. and Kiss, E. W., The shape of congruence lattices. Mem. Amer. Math. Soc. 222(2013), no. 1046. https://doi.org/10.1090/S0065-9266-2012-00667-8Google Scholar
Kozik, M., A finite set of functions with an EXPTIME-complete composition problem. Theoret. Comput. Sci. 407(2008), 330–341. https://doi.org/10.1016/j.tcs.2008.06.057CrossRefGoogle Scholar
Mayr, P., The subpower membership problem for Mal’cev algebras. Internat. J. Algebra Comput. 22(2012), 1250075. https://doi.org/10.1142/S0218196712500750CrossRefGoogle Scholar
Olšák, M., The weakest nontrivial idempotent equations. Bull. London Math. Soc. 49(2017), 1028–1047. https://doi.org/10.1112/blms.12097CrossRefGoogle Scholar
Valeriote, M. and Willard, R., Idempotent n-permutable varieties. Bull. Lond. Math. Soc. 46(2014), 870–880. https://doi.org/10.1112/blms/bdu044CrossRefGoogle Scholar