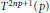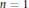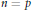Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Theriault, Stephen
2015.
Anick's space and Hopf invariants.
Topology and its Applications,
Vol. 196,
Issue. ,
p.
183.
Amelotte, Steven
2020.
The fibre of the degree 3 map, Anick spaces and the double suspension.
Proceedings of the Edinburgh Mathematical Society,
Vol. 63,
Issue. 3,
p.
830.
Selick, Paul
and
Theriault, Stephen
2022.
Comparing constructions of the classifying space for the fibre of the double suspension.
Topology and its Applications,
Vol. 317,
Issue. ,
p.
108163.