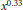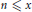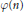Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Banks, William D.
and
Luca, Florian
2011.
Power Totients with Almost Primes.
Integers,
Vol. 11,
Issue. 3,
Banks, William D.
2012.
Carmichael numbers with a totient of the form a 2 + nb 2.
Monatshefte für Mathematik,
Vol. 167,
Issue. 2,
p.
157.
FREIBERG, TRISTAN
2012.
PRODUCTS OF SHIFTED PRIMES SIMULTANEOUSLY TAKING PERFECT POWER VALUES.
Journal of the Australian Mathematical Society,
Vol. 92,
Issue. 2,
p.
145.
Banks, William
Finch, Carrie
Luca, Florian
Pomerance, Carl
and
Stănică, Pantelimon
2014.
Sierpiński and Carmichael numbers.
Transactions of the American Mathematical Society,
Vol. 367,
Issue. 1,
p.
355.