Published online by Cambridge University Press: 20 November 2018
Let 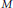 $M$ be a finite module over a commutative noetherian ring
$M$ be a finite module over a commutative noetherian ring  $R$. For ideals
$R$. For ideals  $\mathfrak{a}$ and
$\mathfrak{a}$ and  $\mathfrak{b}$ of
$\mathfrak{b}$ of  $R$, the relations between cohomological dimensions of
$R$, the relations between cohomological dimensions of 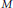 $M$ with respect to
$M$ with respect to  $\mathfrak{a},\,\mathfrak{b},\,\mathfrak{a}\,\cap \,\mathfrak{b}$ and
$\mathfrak{a},\,\mathfrak{b},\,\mathfrak{a}\,\cap \,\mathfrak{b}$ and 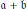 $\mathfrak{a}\,+\,\mathfrak{b}$ are studied. When
$\mathfrak{a}\,+\,\mathfrak{b}$ are studied. When  $R$ is local, it is shown that
$R$ is local, it is shown that 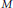 $M$ is generalized Cohen–Macaulay if there exists an ideal
$M$ is generalized Cohen–Macaulay if there exists an ideal  $\mathfrak{a}$ such that all local cohomology modules of
$\mathfrak{a}$ such that all local cohomology modules of 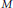 $M$ with respect to
$M$ with respect to  $\mathfrak{a}$ have finite lengths. Also, when
$\mathfrak{a}$ have finite lengths. Also, when  $r$ is an integer such that
$r$ is an integer such that  $0\,\le \,r\,<\,{{\dim}_{R}}(M)$, any maximal element q of the non-empty set of ideals {
$0\,\le \,r\,<\,{{\dim}_{R}}(M)$, any maximal element q of the non-empty set of ideals {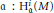 $\mathfrak{a}\,:\,\text{H}_{\mathfrak{a}}^{i}(M)$ is not artinian for some
$\mathfrak{a}\,:\,\text{H}_{\mathfrak{a}}^{i}(M)$ is not artinian for some  $i$,
$i$, 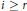 $i\,\ge \,r$} is a prime ideal, and all Bass numbers of
$i\,\ge \,r$} is a prime ideal, and all Bass numbers of 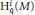 $\text{H}_{\mathfrak{q}}^{i}(M)$ are finite for all
$\text{H}_{\mathfrak{q}}^{i}(M)$ are finite for all 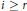 $i\,\ge \,r$.
$i\,\ge \,r$.