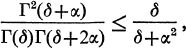Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kabe, D. G.
1978.
An Inequality for Gamma Functions.
Canadian Mathematical Bulletin,
Vol. 21,
Issue. 2,
p.
243.
Merkle, M.
2005.
Gurland's ratio for the gamma function.
Computers & Mathematics with Applications,
Vol. 49,
Issue. 2-3,
p.
389.
2012.
Zeta and q-Zeta Functions and Associated Series and Integrals.
p.
603.
Yang, Zhen-Hang
Qian, Wei-Mao
Chu, Yu-Ming
and
Zhang, Wen
2017.
On rational bounds for the gamma function.
Journal of Inequalities and Applications,
Vol. 2017,
Issue. 1,