Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-23T05:34:27.199Z Has data issue: false hasContentIssue false
  • English
  • Français

An Endpoint Alexandrov Bakelman Pucci Estimate in the Plane

Part of: Partial differential equations Manifolds Classical measure theory

Published online by Cambridge University Press:  11 January 2019

Stefan Steinerberger
Stefan Steinerberger*
Affiliation:
Department of Mathematics, Yale University, New Haven, CT 06511, USA Email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The classical Alexandrov–Bakelman–Pucci estimate for the Laplacian states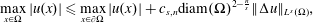

$$\begin{eqnarray}\max _{x\in \unicode[STIX]{x03A9}}|u(x)|\leqslant \max _{x\in \unicode[STIX]{x2202}\unicode[STIX]{x03A9}}|u(x)|+c_{s,n}\text{diam}(\unicode[STIX]{x03A9})^{2-\frac{n}{s}}\Vert \unicode[STIX]{x0394}u\Vert _{L^{s}(\unicode[STIX]{x03A9})},\end{eqnarray}$$
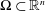where $\unicode[STIX]{x03A9}\subset \mathbb{R}^{n}$, 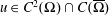$u\in C^{2}(\unicode[STIX]{x03A9})\cap C(\overline{\unicode[STIX]{x03A9}})$ and 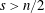$s>n/2$. The inequality fails for 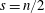$s=n/2$. A Sobolev embedding result of Milman and Pustylnik, originally phrased in a slightly different context, implies an endpoint inequality: if 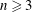$n\geqslant 3$ and 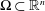$\unicode[STIX]{x03A9}\subset \mathbb{R}^{n}$ is bounded, then
$$\begin{eqnarray}\max _{x\in \unicode[STIX]{x03A9}}|u(x)|\leqslant \max _{x\in \unicode[STIX]{x2202}\unicode[STIX]{x03A9}}|u(x)|+c_{n}\Vert \unicode[STIX]{x0394}u\Vert _{L^{\frac{n}{2},1}(\unicode[STIX]{x03A9})},\end{eqnarray}$$
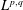 where $L^{p,q}$ is the Lorentz space refinement of 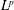$L^{p}$. This inequality fails for 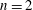$n=2$, and we prove a sharp substitute result: there exists 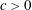$c>0$ such that for all 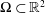$\unicode[STIX]{x03A9}\subset \mathbb{R}^{2}$ with finite measure,
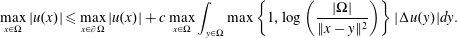$$\begin{eqnarray}\max _{x\in \unicode[STIX]{x03A9}}|u(x)|\leqslant \max _{x\in \unicode[STIX]{x2202}\unicode[STIX]{x03A9}}|u(x)|+c\max _{x\in \unicode[STIX]{x03A9}}\int _{y\in \unicode[STIX]{x03A9}}\max \left\{1,\log \left(\frac{|\unicode[STIX]{x03A9}|}{\Vert x-y\Vert ^{2}}\right)\right\}|\unicode[STIX]{x0394}u(y)|dy.\end{eqnarray}$$
 This is somewhat dual to the classical Trudinger–Moser inequality; we also note that it is sharper than the usual estimates given in Orlicz spaces; the proof is rearrangement-free. The Laplacian can be replaced by any uniformly elliptic operator in divergence form.

Keywords

Alexandrov–Bakelman–Pucci estimatesecond order Sobolev inequalityTrudinger-Moser inequality

MSC classification

Primary: 35A23: Inequalities involving derivatives and differential and integral operators, inequalities for integrals
Secondary: 35B50: Maximum principles 28A75: Length, area, volume, other geometric measure theory 49Q20: Variational problems in a geometric measure-theoretic setting
Type
Article
Information
Canadian Mathematical Bulletin , Volume 62 , Issue 3 , September 2019 , pp. 643 - 651
Copyright
© Canadian Mathematical Society 2018 

References

Adams, D., A sharp inequality of J. Moser for higher order derivatives . Ann. of Math. 128(1988), 385–398. https://doi.org/10.2307/1971445.Google Scholar
Aleksandrov, A. D., Certain estimates for the Dirichlet problem . Dokl. Akad. Nauk SSSR 134(1961), 1001–1004; transl. Soviet Math. Dokl. 1 (1961), 1151–1154.Google Scholar
Aleksandrov, A. D., Uniqueness conditions and bounds for the solution of the Dirichlet problem . Vestnik Leningrad. Univ. Ser. Mat. Meh. Astronom. 18(1963), 5–29.Google Scholar
Aleksandrov, A. D., The impossibility of general estimates for solutions and of uniqueness for linear equations with norms weaker than in L n . Vestnik Leningrad Univ. 21(1966), 5–10. Amer. Math. Soc. Translations 68(1968), 162–168.Google Scholar
Aronson, D. G., Non-negative solutions of linear parabolic equations . Ann. Scuola. Norm. Sup. Pisa 22(1968), 607–694.Google Scholar
Astala, K., Iwaniec, T., and Martin, G., Pucci’s conjecture and the Alexandrov inequality for elliptic PDEs in the plane . J. Reine Angew. Math. 591(2006), 49–74. https://doi.org/10.1515/CRELLE.2006.014.Google Scholar
Bakelman, I. J., On the theory of quasilinear elliptic equations . Sibirsk. Mat. Z̆. 2(1961), 179–186.Google Scholar
Bastero, J., Milman, M., and Ruiz, F., A note on L (∞, q) spaces and Sobolev embeddings . Indiana Univ. Math. J. 52(2003), 1215–1230. https://doi.org/10.1512/iumj.2003.52.2364.Google Scholar
Biswas, A and Lörinczi, J., Maximum principles and Aleksandrov-Bakelman-Pucci type estimates for non-local Schrödinger equations with exterior conditions. 2017. arxiv:1711.09267.Google Scholar
Brezis, H. and Wainger, S., A note on limiting cases of Sobolev embeddings and convolution inequalities . Comm. Partial Differential Equations 5(1980), 773–789. https://doi.org/10.1080/03605308008820154.Google Scholar
Cabré, X., On the Alexandroff-Bakelman-Pucci estimate and the reversed Hölder inequality for solutions of elliptic and parabolic equations . Comm. Pure Appl. Math. 48(1995), 539–570. https://doi.org/10.1002/cpa.3160480504.Google Scholar
Cabré, X., Isoperimetric, Sobolev, and eigenvalue inequalities via the Alexandroff-Bakelman-Pucci method: a survey . Chin. Ann. Math. Ser. B 38(2017), 201–214. https://doi.org/10.1007/s11401-016-1067-0.Google Scholar
Caffarelli, L. and Cabré, X., Fully nonlinear elliptic equations. American Mathematical Society Colloquium Publications, 43, American Mathematical Society, Providence, RI, 1995. https://doi.org/10.1090/coll/043.Google Scholar
Cassani, D., Ruf, B., and Tarsi, C., Best constants in a borderline case of second-order Moser type inequalities . Ann. Inst. H. Poincaré Anal. Non Linéaire 27(2010), 73–93. https://doi.org/10.1016/j.anihpc.2009.07.006.Google Scholar
Cianchi, A., Symmetrization and second-order Sobolev inequalities . Ann. Mat. Pura Appl. 183(2004), 45–77. https://doi.org/10.1007/s10231-003-0080-6.Google Scholar
Cianchi, A. and Maz’ya, V., Sobolev inequalities in arbitrary domains . Adv. Math. 293(2016), 644–696. https://doi.org/10.1016/j.aim.2016.02.012.Google Scholar
Gilbarg, D. and Trudinger, N., Elliptic partial differential equations of second order . Grundlehren der Mathematischen Wissenschaften . Springer, 1983. https://doi.org/10.1007/978-3-642-61798-0.Google Scholar
Grafakos, L., Classical Fourier analysis. . Graduate Texts in Mathematics . Springer, New York, 2008.Google Scholar
Han, Q. and Lin, F., Elliptic partial differential equations . Courant Lecture Notes in Mathematics . American Mathematical Society, Providence, RI, 1997.Google Scholar
Jost, J., Partial differential equations. Graduate Texts in Mathematics, 214, Springer-Verlag, New York, 2002.Google Scholar
Lierl, J. and Steinerberger, S., A local Faber-Krahn inequality and applications to Schrodinger’s equation . Comm. Partial Differential Equations 43(2018), 66–81. https://doi.org/10.1080/03605302.2017.1423330.Google Scholar
Milman, M. and Pustylnik, E., On sharp higher order Sobolev embeddings . Comm. Contemp. Math. 6(2004), 495–511. https://doi.org/10.1142/S0219199704001380.Google Scholar
Milman, M., BMO: oscillations, self-improvement, Gagliardo coordinate spaces, and reverse Hardy inequalities. In: Harmonic analysis, partial differential equations, complex analysis, Banach spaces, and operator theory. 1, Assoc. Women Math. Ser. Assoc. Women Math. Ser., 4, Springer, 2016, 233–274.Google Scholar
Milman, M., Addendum to: BMO: oscillations, self improvement, Gagliardo coordinate spaces and reverse Hardy inequalities. 2018. arxiv:1806.08275.Google Scholar
Moser, J., A sharp form of an inequality by N. Trudinger . Indiana Univ. Math. J. 20(1970/71), 1077–1092. https://doi.org/10.1512/iumj.1971.20.20101.Google Scholar
Pérez Làzaro, F., A note on extreme cases of Sobolev embeddings . J. Math. Anal. Appl. 320(2006), 973–982. https://doi.org/10.1016/j.jmaa.2005.07.019.Google Scholar
Pucci, C., Limitazioni per soluzioni di equazioni ellittiche . Ann. Mat. Pura Appl. 74(1966), 15–30. https://doi.org/10.1007/BF02416445.Google Scholar
Pucci, C., Operatori ellittici estremanti . Ann. Mat. Pura. Appl. (4) 72(1966), 141–170. https://doi.org/10.1007/BF02414332.Google Scholar
Rachh, M. and Steinerberger, S., On the location of maxima of solutions of Schroedinger’s equation . Comm. Pure Appl. Math 71(2018), 1109–1122. https://doi.org/10.1002/cpa.21753.Google Scholar
Steinerberger, S., Lower bounds on nodal sets of eigenfunctions via the heat flow . Comm. Partial Differential Equations 39(2014), 2240–2261. https://doi.org/10.1080/03605302.2014.942739.Google Scholar
Talenti, G., Elliptic equations and rearrangements . Ann. Scuola Norm. Sup. Pisa Cl. Sci. (4) 3(1976), 697–718.Google Scholar
Trudinger, N., On imbeddings into Orlicz spaces and some applications . J. Math. Mech. 17(1967), 473–483.Google Scholar
Tso, K., On an Aleksandrov-Bakelman type maximum principle for second-order parabolic equations . Comm. Partial Differential Equations 10(1985), 543–553. https://doi.org/10.1080/03605308508820388.Google Scholar
Xiao, J. and Zhai, Zh., Fractional Sobolev, Moser-Trudinger Morrey-Sobolev inequalities under Lorentz norms. Problems in mathematical analysis . J. Math. Sci. (N.Y.) 166(2010), 357–376. https://doi.org/10.1007/s10958-010-9872-6.Google Scholar