Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-24T16:15:08.976Z Has data issue: false hasContentIssue false
  • English
  • Français

An Asymptotic Bound on the Composition Number of Integer Sums of Squares Formulas

Published online by Cambridge University Press:  20 November 2018

P. Hrubeš ,
A. Wigderson  and
A. Yehudayoff
P. Hrubeš
Affiliation:
School of Mathematics, Institute for Advanced Study, Princeton, NJ 08540, USA e-mail: [email protected] e-mail: [email protected] e-mail: [email protected]
A. Wigderson
Affiliation:
School of Mathematics, Institute for Advanced Study, Princeton, NJ 08540, USA e-mail: [email protected] e-mail: [email protected] e-mail: [email protected]
A. Yehudayoff
Affiliation:
School of Mathematics, Institute for Advanced Study, Princeton, NJ 08540, USA e-mail: [email protected] e-mail: [email protected] e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let ${{\sigma }_{\mathbb{Z}}}\left( k \right)$ be the smallest $n$ such that there exists an identity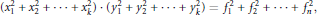

$$\left( x_{1}^{2}\,+\,x_{2}^{2}\,+\,\cdot \cdot \cdot \,+\,x_{k}^{2} \right)\,\cdot \,\left( y_{1}^{2}\,+\,y_{2}^{2}\,+\,\cdot \cdot \cdot \,+\,y_{k}^{2} \right)\,=\,f_{1}^{2}\,+\,f_{2}^{2}\,+\,\cdot \cdot \cdot \,+\,f_{n}^{2},$$
with ${{f}_{1}},...,\,{{f}_{n}}$ being polynomials with integer coefficients in the variables 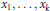${{x}_{1}},...,\,{{x}_{k}}$ and 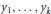${{y}_{1}},...,\,{{y}_{k}}$. We prove that 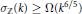${{\sigma }_{\mathbb{Z}}}\left( k \right)\,\ge \,\Omega \left( {{k}^{{6}/{5}\;}} \right)$.

Keywords

11E25composition formulassums of squaresRadon-Hurwitz number
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 56 , Issue 1 , 01 March 2013 , pp. 70 - 79
Copyright
Copyright © Canadian Mathematical Society 2013

References

[1] Hrubeš, P., Wigderson, A., and Yehudayoff, A., Non-commutative circuits and the sum-of-squares problem. J. Amer. Math. Soc. 24((2011), 871–898. http://dx.doi.org/10.1090/S0894-0347-2011-00694-2 Google Scholar
[2] Hurwitz, A., Über die Komposition der quadratischen Formen von beliebig vielen Variabeln. Nach. Ges.Wiss. Göttingen (1898), 309–316.Google Scholar
[3] Hurwitz, A., Über die Komposition der quadratischen Formen. Math. Ann. 88((1923), 1–25. http://dx.doi.org/10.1007/BF01448439 Google Scholar
[4] James, I. M.. On the immersion problem for real projective spaces. Bull. Amer. Math. Soc. 69((1967), 231–238. http://dx.doi.org/10.1090/S0002-9904-1963-10930-1 Google Scholar
[5] Kirkman, T., On pluquatemions, and horaoid products of sums of n squares. Philos. Mag. (ser. 3) 33((1848), 447–459, 494–509.Google Scholar
[6] Lam, K. Y., Some new results on composition of quadratic forms. Invent. Math. 79((1985), 467–474. http://dx.doi.org/10.1007/BF01388517 Google Scholar
[7] Lam, T. Y. and Smith, T., On Yuzvinsky's monomial pairings. Quart. J. Math. Oxford (2) 44((1993), 215–237. http://dx.doi.org/10.1093/qmath/44.2.215 Google Scholar
[8] Pfister, A., Multiplikative quadratische Formen. Arch. Math. 16((1965), 363–370.Google Scholar
[9] Radon, J., Lineare scharen orthogonalen matrizen. Abh. Math. Sem. Univ. Hamburg 1((1922), 2–14.Google Scholar
[10] Shapiro, D. B., Compositions of quadratic forms. de Gruyter Expositions in Mathematics 33, Walter de Gruyter & Co., Berlin, 2000.Google Scholar
[11] Yiu, P., Sums of squares formulae with integer coefficients. Canad. Math. Bull. 30((1987), 318–324. http://dx.doi.org/10.4153/CMB-1987-045-6 Google Scholar
[12] Yiu, P., On the product of two sums of 16 squares as a sum of squares of integral bilinear forms. Quart. J. Math. Oxford (2) 41((1990), 463–500. http://dx.doi.org/10.1093/qmath/41.4.463 Google Scholar
[13] Yuzvinsky, S., A series of monomial pairings. Linear and Multilinear Algebra 15((1984), 19–119. http://dx.doi.org/10.1080/03081088408817582 Google Scholar