No CrossRef data available.
 ${\mathcal{O}}_{N}$
${\mathcal{O}}_{N}$Published online by Cambridge University Press: 03 January 2020
Representations of the Cuntz algebra 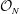 ${\mathcal{O}}_{N}$ are constructed from interval dynamical systems associated with slow continued fraction algorithms introduced by Giovanni Panti. Their irreducible decomposition formulas are characterized by using the modular group action on real numbers, as a generalization of results by Kawamura, Hayashi, and Lascu. Furthermore, a certain symmetry of such an interval dynamical system is interpreted as a covariant representation of the
${\mathcal{O}}_{N}$ are constructed from interval dynamical systems associated with slow continued fraction algorithms introduced by Giovanni Panti. Their irreducible decomposition formulas are characterized by using the modular group action on real numbers, as a generalization of results by Kawamura, Hayashi, and Lascu. Furthermore, a certain symmetry of such an interval dynamical system is interpreted as a covariant representation of the 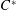 $C^{\ast }$-dynamical system of the “flip-flop” automorphism of
$C^{\ast }$-dynamical system of the “flip-flop” automorphism of  ${\mathcal{O}}_{2}$.
${\mathcal{O}}_{2}$.