No CrossRef data available.
Published online by Cambridge University Press: 08 September 2022
Let f be a primitive Hilbert modular form over F of weight k with coefficient field
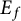 $E_f$
, generated by the Fourier coefficients
$E_f$
, generated by the Fourier coefficients
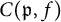 $C(\mathfrak {p}, f)$
for
$C(\mathfrak {p}, f)$
for
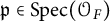 $\mathfrak {p} \in \mathrm {Spec}(\mathcal {O}_F)$
. Under certain assumptions on the image of the residual Galois representations attached to f, we calculate the Dirichlet density of
$\mathfrak {p} \in \mathrm {Spec}(\mathcal {O}_F)$
. Under certain assumptions on the image of the residual Galois representations attached to f, we calculate the Dirichlet density of
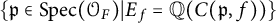 $\{\mathfrak {p} \in \mathrm {Spec}(\mathcal {O}_F)| E_f = \mathbb {Q}(C(\mathfrak {p}, f))\}$
. For
$\{\mathfrak {p} \in \mathrm {Spec}(\mathcal {O}_F)| E_f = \mathbb {Q}(C(\mathfrak {p}, f))\}$
. For
 $k=2$
, we show that those assumptions are satisfied when
$k=2$
, we show that those assumptions are satisfied when
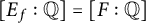 $[E_f:\mathbb {Q}] = [F:\mathbb {Q}]$
is an odd prime. We also study analogous results for
$[E_f:\mathbb {Q}] = [F:\mathbb {Q}]$
is an odd prime. We also study analogous results for
 $F_f$
, the fixed field of
$F_f$
, the fixed field of
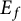 $E_f$
by the set of all inner twists of f. Then, we provide some examples of f to support our results. Finally, we compute the density of
$E_f$
by the set of all inner twists of f. Then, we provide some examples of f to support our results. Finally, we compute the density of
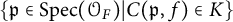 $\{\mathfrak {p} \in \mathrm {Spec}(\mathcal {O}_F)| C(\mathfrak {p}, f) \in K\}$
for fields K with
$\{\mathfrak {p} \in \mathrm {Spec}(\mathcal {O}_F)| C(\mathfrak {p}, f) \in K\}$
for fields K with
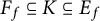 $F_f \subseteq K \subseteq E_f$
.
$F_f \subseteq K \subseteq E_f$
.