Hostname: page-component-669899f699-b58lm Total loading time: 0 Render date: 2025-04-25T13:47:31.576Z Has data issue: false hasContentIssue false
  • English
  • Français

Irreducible modules of modular Lie superalgebras and super version of the first Kac–Weisfeiler conjecture

Part of: Lie algebras and Lie superalgebras

Published online by Cambridge University Press:  11 December 2023

Bin Shu
Bin Shu*
Affiliation:
School of Mathematical Sciences, Ministry of Education Key Laboratory of Mathematics and Engineering Applications & Shanghai Key Laboratory of PMMP, East China Normal University, No. 500 Dongchuan Road, Shanghai 200241, China
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Suppose 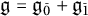$\mathfrak {g}=\mathfrak {g}_{\bar 0}+\mathfrak {g}_{\bar 1}$ is a finite-dimensional restricted Lie superalgebra over an algebraically closed field 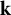$\mathbf {k}$ of characteristic 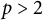$p>2$. In this article, we propose a conjecture for maximal dimensions of irreducible modules over the universal enveloping algebra 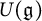$U(\mathfrak {g})$ of 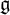$\mathfrak {g}$, as a super generalization of the celebrated first Kac–Weisfeiler conjecture. It is demonstrated that the conjecture holds for all basic classical Lie superalgebras and all completely solvable restricted Lie superalgebras. In this process, we investigate irreducible representations of solvable Lie superalgebras.

Keywords

Modular Lie superalgebrasmaximal dimensions of irreducible modulesthe first Kac–Weisfeiler conjecture

MSC classification

Primary: 17B50: Modular Lie (super)algebras
Secondary: 17B20: Simple, semisimple, reductive (super)algebras 17B30: Solvable, nilpotent (super)algebras
Type
Article
Information
Canadian Mathematical Bulletin , Volume 67 , Issue 3 , September 2024 , pp. 554 - 573
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of Canadian Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

Footnotes

This work is partially supported by the National Natural Science Foundation of China (Grant Nos. 12071136, 11771279, and 12271345), supported in part by Science and Technology Commission of Shanghai Municipality (Grant No. 22DZ2229014).

References

Brundan, J., Modular representations of the supergroup $Q(n)$ , II . Pacific J. Math. 224(2006), 65–90.CrossRefGoogle Scholar
Brundan, J. and Kleshchev, A., Modular representations of the supergroup Q(n), I . J. Algebra 260(2003), 64–98.CrossRefGoogle Scholar
Brundan, J. and Kujawa, J., A new proof of the Mullineux conjecture . J. Algebraic Combin. 18(2003), 13–39.CrossRefGoogle Scholar
Carmeli, C., Caston, L., and Fioresi, R., Mathematical foundations of supersymmetry. Vol. 15, European Mathematical Society, Zürich, 2011.CrossRefGoogle Scholar
Cheng, S.-J., Lam, N., and Wang, W., The Brundan–Kazhdan–Lusztig conjecture for general linear Lie superalgebras . Duke Math. J. 164(2015), no. 4, 617–695.CrossRefGoogle Scholar
Cheng, S.-J., Shu, B., and Wang, W., Modular representations of exceptional supergroups . Math. Z. 291(2019),635–659.CrossRefGoogle Scholar
Fioresi, R. and Gavarini, F., Chevalley supergroups . Mem. Amer. Math. Soc. 215(2012), 1014.Google Scholar
Fioresi, R. and Gavarini, F., Algebraic supergroups with Lie superalgebras of classical type . J. Lie Theory 23(2013), 143–158.Google Scholar
Goodwin, S. M. and Topley, L., Minimal-dimensional representations of reduced enveloping algebras for ${\mathfrak{gl}}_n$ . Compos. Math. 155(2019), 1594–1617.CrossRefGoogle Scholar
Jacobson, N., Lie algebras, Interscience Tracts in Pure and Applied Mathematics, No. 10, Interscience Publishers (a division of John Wiley & Sons, Inc.), New York, 1962.Google Scholar
Kac, V. G., Lie superalgebras . Adv. Math. 26(1977), 8–96.CrossRefGoogle Scholar
Kac, V. G., Review on [17], MR 1345285 (96g: 17007), MathSciNet, Amer. Math. Soc.Google Scholar
Li, Y. and Shu, B., Jantzen filtration of Weyl modules for general linear supergroups . Forum. Math. 35(2023), 1435–1468.CrossRefGoogle Scholar
Liu, W., Wang, S., and Yuan, J., On restricted simple modules of contact Lie superalgebras of odd type . Math. Nachr. 290(2017), 2934–2947.CrossRefGoogle Scholar
Martin, B., Stewart, D., and Topley, L., A proof of the first Kac–Weisfeiler conjecture in large characteristics, with an appendix by T. Akaki . Represent. Theory 23(2019), 278–293.CrossRefGoogle Scholar
Pan, L. and Shu, B., Jantzen filtration and strong linkage principle for modular Lie superalgebras . Forum. Math. 30(2018), 1573–1598.CrossRefGoogle Scholar
Premet, A., Irreducible representations of Lie algebras of reductive groups and the Kac–Weisfeiler conjecture . Invent. Math. 121(1995), 79–117.CrossRefGoogle Scholar
Premet, A. and Skryabin, S., Representations of restricted Lie algebras and families of associative –algebras . J. Reine Angew. Math. 507(1999), 189–218.CrossRefGoogle Scholar
Ren, Y., Shu, B., Yang, F., and Zhang, A., Modular representations of strange classical Lie superalgebras and the first super Kac–Weisfeiler conjecture. Preprint, 2023. arXiv:2307.00483[math.RT] Google Scholar
Schue, J., Representations of solvable Lie $p$ -algebras . J. Algebra 38(1976), 253–267.CrossRefGoogle Scholar
Shu, B. and Wang, W., Modular representations of the ortho-symplectic supergroups . Proc. Lond. Math. Soc. 96(2008), 251–271.CrossRefGoogle Scholar
Shu, B. and Yao, Y., Character formulas for restricted simple modules of the special superalgebras . Math. Nachr. 285(2012), 1107–1116.CrossRefGoogle Scholar
Shu, B. and Zhang, C., Restricted representations of the Witt superalgebras . J. Algebra 324(2010), no. 4, 652–672.CrossRefGoogle Scholar
Shu, B. and Zhang, C., Representations of the restricted Cartan type Lie superalgebra W(m,n,1) . Algebr. Represent. Theory 14(2011), 463–481.CrossRefGoogle Scholar
Shu, B. and Zheng, L., On Lie superalgebras of algebraic supergroups . Algebra Colloq. 16(2009), 361–370.CrossRefGoogle Scholar
Strade, H. and Farnsteiner, R., Modular Lie algebras and their representations, Marcel Dekker, New York, 1988.Google Scholar
Topley, L., A non-restricted counterexample to the first Kac–Weisfeiler conjecture . Proc. Amer. Math. Soc. 45(2016), 1937–1942.CrossRefGoogle Scholar
Wang, S., Yuan, J., and Liu, W., Restricted Kac modules for special contact Lie superalgebras of odd type . Front. Math. China 15(2020), 419–434.CrossRefGoogle Scholar
Wang, W. and Zhao, L., Representations of Lie superalgebras in prime characteristic I . Proc. Lond. Math. Soc. (3) 99(2009), 145–167.CrossRefGoogle Scholar
Wang, W. and Zhao, L., Representations of Lie superalgebras in prime characteristic II: The queer series . J. Pure Appl. Algebra 215(2011), 2515–2532.CrossRefGoogle Scholar
Weisfeiler, B. J. and Kac, V. G., The irreducible representations of Lie $p$ -algebras . Funcional. Anal. i Priložen. 5(1971), 28–36.Google Scholar
Yao, Y., On restricted representations of the extended special type Lie superalgebra $\overline{S}(m,n,1)$ . Monatsh. Math. 170(2013), 239–255.CrossRefGoogle Scholar
Yao, Y., Irreducible representations of the classical Lie superalgebra of type $A\left(0,n\right)$ in prime characteristic . Monatsh. Math. 172(2013), 207–231.CrossRefGoogle Scholar
Yao, Y., Character formulas for a class of simple restricted modules over the simple Lie superalgebras of Witt type . Chinese Ann. Math. Ser. B 41(2020), 49–60.CrossRefGoogle Scholar
Yao, Y. and Shu, B., Restricted representations of Lie superalgebras of Hamiltonian type . Algebr. Represent. Theory 16(2013), 615–632.CrossRefGoogle Scholar
Yao, Y. and Shu, B., A note on restricted representations of the Witt superalgebras . Chinese Ann. Math. Ser. B 34(2013), 921–926.CrossRefGoogle Scholar
Yuan, J. and Liu, W., Restricted Kac modules of Hamiltonian Lie superalgebras of odd type . Monatsh. Math. 178(2015), 473–488.CrossRefGoogle Scholar
Zassenhaus, H., The representations of Lie algebras of prime characteristic . Proc. Glasgow Math. Assoc. 2(1954), 1–36.CrossRefGoogle Scholar
Zeng, Y. and Shu, B., On Kac–Weisfeiler modules for general and special linear Lie superalgebras . Israel J. Math. 214(2016), 471–490.CrossRefGoogle Scholar
Zeng, Y. and Shu, B., Finite W-superalgebras and dimensional lower bounds for the representations of basic Lie superalgebras . Publ. Res. Inst. Math. Sci. 53(2017), 1–63.CrossRefGoogle Scholar
Zhang, C., On the simple modules for the restricted Lie superalgebra $\mathfrak{sl}(n|1)$ . J. Pure Appl. Algebra 213(2009), 756–765.CrossRefGoogle Scholar
Zhao, L., Representations of Lie superalgebras in prime characteristic, III . Pacific J. Math. 248(2010), 493–510.CrossRefGoogle Scholar
Zheng, L. and Shu, B., Representations of $\mathfrak{gl}(m|n)$ and infinitesimal subgroups of $GL(m|n)$ . Chinese Ann. Math. Ser. A 31(2010), 129–142.Google Scholar