Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Tewodrose, David
2021.
A survey on spectral embeddings and their application in data analysis.
Séminaire de théorie spectrale et géométrie,
Vol. 35,
Issue. ,
p.
197.
Milman, Emanuel
2021.
The Quasi Curvature‐Dimension Condition with Applications to Sub‐Riemannian Manifolds.
Communications on Pure and Applied Mathematics,
Vol. 74,
Issue. 12,
p.
2628.
Stefani, Giorgio
2022.
Generalized Bakry–Émery Curvature Condition and Equivalent Entropic Inequalities in Groups.
The Journal of Geometric Analysis,
Vol. 32,
Issue. 4,
Lučić, Danka
and
Pasqualetto, Enrico
2022.
Gamma-convergence of Cheeger energies with respect to increasing distances.
Journal of Mathematical Analysis and Applications,
Vol. 515,
Issue. 1,
p.
126415.
Górny, Wojciech
and
Mazón, José M.
2022.
On the p-Laplacian evolution equation in metric measure spaces.
Journal of Functional Analysis,
Vol. 283,
Issue. 8,
p.
109621.
Le Donne, Enrico
Lučić, Danka
and
Pasqualetto, Enrico
2023.
Universal Infinitesimal Hilbertianity of Sub-Riemannian Manifolds.
Potential Analysis,
Vol. 59,
Issue. 1,
p.
349.
Fornasier, Massimo
Savaré, Giuseppe
and
Sodini, Giacomo Enrico
2023.
Density of subalgebras of Lipschitz functions in metric Sobolev spaces and applications to Wasserstein Sobolev spaces.
Journal of Functional Analysis,
Vol. 285,
Issue. 11,
p.
110153.
Lučić, Danka
Pasqualetto, Enrico
and
Rajala, Tapio
2023.
Non-Hilbertian tangents to Hilbertian spaces.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 153,
Issue. 3,
p.
811.
Górny, Wojciech
and
Mazón, J. M.
2024.
The Anzellotti–Gauss–Green formula and least gradient functions in metric measure spaces.
Communications in Contemporary Mathematics,
Vol. 26,
Issue. 06,
Ambrosio, Luigi
Ikonen, Toni
Lučić, Danka
and
Pasqualetto, Enrico
2024.
Metric Sobolev Spaces I: Equivalence of Definitions.
Milan Journal of Mathematics,
Vol. 92,
Issue. 2,
p.
255.
Górny, Wojciech
and
Mazón, José M.
2024.
The Neumann and Dirichlet problems for the total variation flow in metric measure spaces.
Advances in Calculus of Variations,
Vol. 17,
Issue. 1,
p.
131.
 $(M,g,\unicode[STIX]{x1D707})$, i.e., a Riemannian manifold
$(M,g,\unicode[STIX]{x1D707})$, i.e., a Riemannian manifold 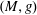 $(M,g)$ endowed with a generic non-negative Radon measure
$(M,g)$ endowed with a generic non-negative Radon measure  $\unicode[STIX]{x1D707}$, is infinitesimally Hilbertian, which means that its associated Sobolev space
$\unicode[STIX]{x1D707}$, is infinitesimally Hilbertian, which means that its associated Sobolev space 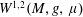 $W^{1,2}(M,g,\unicode[STIX]{x1D707})$ is a Hilbert space.
$W^{1,2}(M,g,\unicode[STIX]{x1D707})$ is a Hilbert space.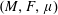 $(M,F,\unicode[STIX]{x1D707})$ can be isometrically embedded into the space of all measurable sections of the tangent bundle of
$(M,F,\unicode[STIX]{x1D707})$ can be isometrically embedded into the space of all measurable sections of the tangent bundle of 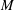 $M$ that are
$M$ that are 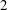 $2$-integrable with respect to
$2$-integrable with respect to 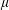 $\unicode[STIX]{x1D707}$.
$\unicode[STIX]{x1D707}$.

