Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-24T01:53:29.842Z Has data issue: false hasContentIssue false
  • English
  • Français

Geography of simply connected nonspin symplectic 4-manifolds with positive signature. II

Part of: Differential topology

Published online by Cambridge University Press:  10 July 2020

Anar Akhmedov  and
B. Doug Park
Anar Akhmedov
Affiliation:
School of Mathematics, University of Minnesota, Minneapolis, MN55455, USA e-mail: [email protected]
B. Doug Park*
Affiliation:
Department of Pure Mathematics, University of Waterloo, Waterloo, ONN2L 3G1, Canada
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Building upon our earlier work with M. C. Hughes, we construct many new smooth structures on closed simply connected nonspin $4$ -manifolds with positive signature. We also provide numerical and asymptotic upper bounds on the function 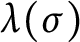$\lambda (\sigma )$ that was defined in our earlier work.

Keywords

Symplectic 4-manifoldgeographyknot surgerysymplectic normal sum

MSC classification

Secondary: 57R55: Differentiable structures
Type
Article
Information
Canadian Mathematical Bulletin , Volume 64 , Issue 2 , June 2021 , pp. 418 - 428
Check for updates
Copyright
© Canadian Mathematical Society 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The first author was partially supported by a Simons Research Fellowship and Collaboration Grant for Mathematicians from the Simons Foundation. The second author was partially supported by an NSERC discovery grant.

References

Akhmedov, A., Hughes, M. C., and Park, B. D., Geography of simply connected nonspin symplectic $\;4$ -manifolds with positive signature . Pacific J. Math. 261(2013), 257–282. https://doi.org/10.2140/pjm.2013.261.257 CrossRefGoogle Scholar
Akhmedov, A. and Park, B. D., New symplectic $\;4$ -manifolds with nonnegative signature . J. Gökova Geom. Topol. GGT 2(2008), 1–13.Google Scholar
Akhmedov, A. and Park, B. D., Geography of simply connected spin symplectic $\;4$ -manifolds. Math. Res. Lett. 17(2010), 483–492. https://doi.org/10.4310/MRL.2010.v17.n3.a8 CrossRefGoogle Scholar
Akhmedov, A. and Park, B. D., Geography of simply connected spin symplectic $\;4$ -manifolds. II. C. R. Math. Acad. Sci. Paris 357(2019), 296–298. https://doi.org/10.1016/j.crma.2019.02.002 CrossRefGoogle Scholar
Akhmedov, A. and Sakallı, S., On the geography of simply connected nonspin symplectic $\;4$ -manifolds with nonnegative signature. Topology Appl. 206(2016), 24–45. https://doi.org/10.1016/j.topol.2016.03.026 CrossRefGoogle Scholar
Bauer, I. C. and Catanese, F., A volume maximizing canonical surface in $\;3$ -space. Comment. Math. Helv. 83(2008), 387–406. https://doi.org/10.4171/CMH/129 CrossRefGoogle Scholar
Burde, G., Alexanderpolynome Neuwirthscher Knoten. Topology 5(1966), 321–330. https://doi.org/10.1016/0040-9383(66)90023-1 CrossRefGoogle Scholar
Catanese, F. and Dettweiler, M., Vector bundles on curves coming from variation of Hodge structures. Int. J. Math. 27(2016), 1640001. https://doi.org/10.1142/S0129167X16400012 CrossRefGoogle Scholar
Fintushel, R. and Stern, R. J., Knots, links and $\;4$ -manifolds. Invent. Math. 134(1998), 363–400. https://doi.org/10.1007/s002220050268 CrossRefGoogle Scholar
Fomenko, A. T. and Matveev, S. V., Algorithmic and computer methods for three-manifolds. In: Mathematics and its applications, 425, Kluwer Academic Publishers, Dordrecht, Netherlands, 1997. https://doi.org/10.1007/978-94-017-0699-5 Google Scholar
Freedman, M. H., The topology of four-dimensional manifolds. J. Differ. Geom. 17(1982), 357–453.Google Scholar
Gompf, R. E., A new construction of symplectic manifolds. Ann. Math. 142(1995), 527–595. https://doi.org/10.2307/2118554 CrossRefGoogle Scholar
Gompf, R. E. and Mrowka, T. S., Irreducible $\;4$ -manifolds need not be complex. Ann. Math. 138(1993), 61–111. https://doi.org/10.2307/2946635 CrossRefGoogle Scholar
Hamilton, M. J. D. and Kotschick, D., Minimality and irreducibility of symplectic four-manifolds. Int. Math. Res. Not. 2006(2006), 35032. https://doi.org/10.1155/IMRN/2006/35032 Google Scholar
Kotschick, D., The Seiberg-Witten invariants of symplectic four-manifolds (after C. H. Taubes). Séminaire Bourbaki 241(1997), 195–220.Google Scholar
Lee, J., Lönne, M., and Rollenske, S., Double Kodaira fibrations with small signature. Int. J. Math. 31(2020), no. 7, 2050052. https://doi.org/10.1142/S0129167X20500524 CrossRefGoogle Scholar
McCarthy, J. D. and Wolfson, J. G., Symplectic normal connect sum. Topology 33(1994), 729–764. https://doi.org/10.1016/0040-9383(94)90006-X CrossRefGoogle Scholar
Pardini, R., Abelian covers of algebraic varieties. J. Reine Angew. Math. 417(1991), 191–213. https://doi.org/10.1515/cr11.1991.417.191 Google Scholar
Usher, M., Minimality and symplectic sums . Int. Math. Res. Not. 2006(2006), 49857. https://doi.org/10.1155/IMRN/2006/49857 Google Scholar