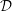 ${\mathcal{D}}$-Einstein Real Hypersurfaces in
${\mathcal{D}}$-Einstein Real Hypersurfaces in 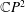 $\mathbb{C}P^{2}$ and
$\mathbb{C}P^{2}$ and 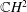 $\mathbb{C}H^{2}$
$\mathbb{C}H^{2}$Published online by Cambridge University Press: 27 February 2020
In this paper we obtain some new characterizations of pseudo-Einstein real hypersurfaces in 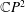 $\mathbb{C}P^{2}$ and
$\mathbb{C}P^{2}$ and 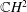 $\mathbb{C}H^{2}$. More precisely, we prove that a real hypersurface in
$\mathbb{C}H^{2}$. More precisely, we prove that a real hypersurface in 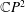 $\mathbb{C}P^{2}$ or
$\mathbb{C}P^{2}$ or 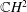 $\mathbb{C}H^{2}$ with constant mean curvature is generalized
$\mathbb{C}H^{2}$ with constant mean curvature is generalized  ${\mathcal{D}}$-Einstein with constant coefficient if and only if it is pseudo-Einstein. We prove that a real hypersurface in
${\mathcal{D}}$-Einstein with constant coefficient if and only if it is pseudo-Einstein. We prove that a real hypersurface in  $\mathbb{C}P^{2}$ with constant scalar curvature is generalized
$\mathbb{C}P^{2}$ with constant scalar curvature is generalized 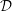 ${\mathcal{D}}$-Einstein with constant coefficient if and only if it is pseudo-Einstein.
${\mathcal{D}}$-Einstein with constant coefficient if and only if it is pseudo-Einstein.
This work was supported by the Key Scientific Research Program in Universities of Henan Province (No. 20A110023) and the Fostering Foundation of National Foundation in Henan Normal University (No. 2019PL22).