Hostname: page-component-6587cd75c8-4pd2k Total loading time: 0 Render date: 2025-04-24T03:34:36.614Z Has data issue: false hasContentIssue false
  • English
  • Français

Compatibility of Kazhdan and Brauer homomorphism

Part of: Discontinuous groups and automorphic forms Lie groups

Published online by Cambridge University Press:  22 November 2024

Sabyasachi Dhar Open the ORCID record for Sabyasachi Dhar [Opens in a new window]
Sabyasachi Dhar*
Affiliation:
Department of Mathematics and Statistics, Indian Institute of Technology Kanpur, U.P. 208016, India
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let G be a split connected reductive group defined over 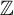$\mathbb {Z}$. Let F and 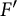$F'$ be two non-Archimedean m-close local fields, where m is a positive integer. D. Kazhdan gave an isomorphism between the Hecke algebras 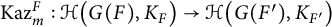$\mathrm {Kaz}_m^F :\mathcal {H}\big (G(F),K_F\big ) \rightarrow \mathcal {H}\big (G(F'),K_{F'}\big )$, where $K_F$ and $K_{F'}$ are the mth usual congruence subgroups of $G(F)$ and 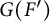$G(F')$, respectively. On the other hand, if 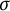$\sigma $ is an automorphism of G of prime order l, then we have Brauer homomorphism 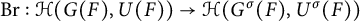$\mathrm {Br}:\mathcal {H}(G(F),U(F))\rightarrow \mathcal {H}(G^\sigma (F),U^\sigma (F))$, where $U(F)$ and 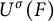$U^\sigma (F)$ are compact open subgroups of 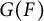$G(F)$ and $G^\sigma (F),$ respectively. In this article, we study the compatibility between these two maps in the local base change setting. Further, an application of this compatibility is given in the context of linkage – which is the representation theoretic version of Brauer homomorphism.

Keywords

Hecke algebraKazhdan isomorphismBrauer homomorphismTate cohomologyLinkageClose local fields

MSC classification

Primary: 11F70: Representation-theoretic methods; automorphic representations over local and global fields 22E50: Representations of Lie and linear algebraic groups over local fields
Type
Article
Information
Canadian Mathematical Bulletin , Volume 68 , Issue 1 , March 2025 , pp. 19 - 43
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press on behalf of Canadian Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Allan, N. D., Hecke rings of congruence subgroups . Bull. Amer. Math. Soc. 78(1972), 541–545.CrossRefGoogle Scholar
Badulescu, A. I., Correspondance de Jacquet–Langlands pour les corps locaux de caractéristique non nulle . Ann. Sci. École Norm. Sup. (4) 35(2002), no. 5, 695–747.Google Scholar
Bernstein, J. N., Le “centre” de Bernstein . In: Deligne, P. (ed.), Representations of reductive groups over a local field, Travaux en Cours, Hermann, Paris, 1984, pp. 1–32.Google Scholar
Badulescu, A. I., Henniart, G., Lemaire, B., and Sécherre, V., Sur le dual unitaire de $\mathrm{GL}_r(D)$ . Amer. J. Math. 132(2010), no. 5, 1365–1396.CrossRefGoogle Scholar
Bernstein, I. N. and Zelevinsky, A. V., Induced representations of reductive $p$ -adic groups. I . Ann. Sci. École Norm. Sup. (4) 10(1977), no. 4, 441–472.CrossRefGoogle Scholar
Deligne, P., Les corps locaux de caractéristique $p$ , limites de corps locaux de caractéristique  $0$ . In: Representations of reductive groups over a local field, Travaux en Cours, Hermann, Paris, 1984, pp. 119–157.Google Scholar
Dat, J.-F., Helm, D., Kurinczuk, R., and Moss, G., Finiteness for hecke algebras of $p$ -adic groups . J. Amer. Math. Soc. 37(2024), no. 3, 929–949.CrossRefGoogle Scholar
Feng, T., Smith theory and cyclic base change functoriality, Forum of Mathematics, Pi, 12, Cambridge University Press, Cambridge, 2024, p. e1.CrossRefGoogle Scholar
Ganapathy, R., The local langlands correspondence for gsp 4 over local function fields . Amer. J. Math. 137(2015), 1441–1534.CrossRefGoogle Scholar
Ganapathy, R., A Hecke algebra isomorphism over close local fields . Pacific J. Math. 319(2022), no. 2, 307–332.CrossRefGoogle Scholar
Kazhdan, D., Representations of groups over close local fields . J. Analyse Math. 47(1986), 175–179.CrossRefGoogle Scholar
Kaletha, T. and Prasad, G., Bruhat–Tits theory—a new approach, New Mathematical Monographs, 44, Cambridge University Press, Cambridge, 2023.CrossRefGoogle Scholar
Serre, J.-P., Cohomologie galoisienne. 5th ed., Lecture Notes in Mathematics, Vol. 5, Springer-Verlag, Berlin-New York, 1973, vii+217 pp.CrossRefGoogle Scholar
Treumann, D. and Venkatesh, A., Functoriality, Smith theory, and the Brauer homomorphism . Ann. of Math. (2) 183(2016), no. 1, 177–228.CrossRefGoogle Scholar
Vignéras, M.-F., Représentations $l$ -modulaires d’un groupe réductif $p\!$ -adique avec $l\ne p$ , Progress in Mathematics, 137, Birkhäuser, Boston, MA, 1996.Google Scholar