Published online by Cambridge University Press: 26 June 2023
In this article, we study the Bohr operator for the operator-valued subordination class  $S(f)$ consisting of holomorphic functions subordinate to f in the unit disk
$S(f)$ consisting of holomorphic functions subordinate to f in the unit disk 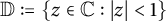 $\mathbb {D}:=\{z \in \mathbb {C}: |z|<1\}$, where
$\mathbb {D}:=\{z \in \mathbb {C}: |z|<1\}$, where 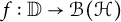 $f:\mathbb {D} \rightarrow \mathcal {B}(\mathcal {H})$ is holomorphic and
$f:\mathbb {D} \rightarrow \mathcal {B}(\mathcal {H})$ is holomorphic and 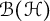 $\mathcal {B}(\mathcal {H})$ is the algebra of bounded linear operators on a complex Hilbert space
$\mathcal {B}(\mathcal {H})$ is the algebra of bounded linear operators on a complex Hilbert space  $\mathcal {H}$. We establish several subordination results, which can be viewed as the analogs of a couple of interesting subordination results from scalar-valued settings. We also obtain a von Neumann-type inequality for the class of analytic self-mappings of the unit disk
$\mathcal {H}$. We establish several subordination results, which can be viewed as the analogs of a couple of interesting subordination results from scalar-valued settings. We also obtain a von Neumann-type inequality for the class of analytic self-mappings of the unit disk 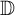 $\mathbb {D}$ which fix the origin. Furthermore, we extensively study Bohr inequalities for operator-valued polyanalytic functions in certain proper simply connected domains in
$\mathbb {D}$ which fix the origin. Furthermore, we extensively study Bohr inequalities for operator-valued polyanalytic functions in certain proper simply connected domains in 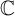 $\mathbb {C}$. We obtain Bohr radius for the operator-valued polyanalytic functions of the form
$\mathbb {C}$. We obtain Bohr radius for the operator-valued polyanalytic functions of the form 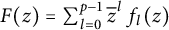 $F(z)= \sum _{l=0}^{p-1} \overline {z}^l \, f_{l}(z) $, where
$F(z)= \sum _{l=0}^{p-1} \overline {z}^l \, f_{l}(z) $, where 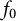 $f_{0}$ is subordinate to an operator-valued convex biholomorphic function, and operator-valued starlike biholomorphic function in the unit disk
$f_{0}$ is subordinate to an operator-valued convex biholomorphic function, and operator-valued starlike biholomorphic function in the unit disk 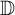 $\mathbb {D}$.
$\mathbb {D}$.
The first-named author is supported by SERB-CRG, and the second-named author is supported by the Institute Postdoctoral Fellowship of IIT Bombay, India.