No CrossRef data available.
Article contents
Asymptotic first boundary value problem for holomorphic functions of several complex variables
Published online by Cambridge University Press: 18 May 2021
Abstract
In 1955, Lehto showed that, for every measurable function 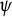 $\psi $
on the unit circle
$\psi $
on the unit circle 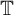 ${\mathbb T}$
, there is a function f holomorphic in the unit disc
${\mathbb T}$
, there is a function f holomorphic in the unit disc 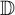 ${{\mathbb D}}$
, having
${{\mathbb D}}$
, having 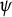 $\psi $
as radial limit a.e. on
$\psi $
as radial limit a.e. on 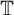 ${\mathbb T}$
. We consider an analogous boundary value problem, where the unit disc is replaced by a Stein domain on a complex manifold and radial approach to a boundary point p is replaced by (asymptotically) total approach to p.
${\mathbb T}$
. We consider an analogous boundary value problem, where the unit disc is replaced by a Stein domain on a complex manifold and radial approach to a boundary point p is replaced by (asymptotically) total approach to p.
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2021
Footnotes
The present research was supported by NSERC (Canada) grant RGPIN-2016-04107.



