Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-11-26T17:43:10.609Z Has data issue: false hasContentIssue false
  • English
  • Français

Wallman Compactification and Representation

Published online by Cambridge University Press:  20 November 2018

Shankar Hegde
Shankar Hegde*
Affiliation:
Northern Illinois University, DeKalb, Illinois
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let X be any set and A be a uniformly closed algebra of bounded real valued functions on X which contains the constants and separates the points. For a lattice ℒ of subsets of X (we assume throughout that ∅ and X belong to ℒ), let MR(ℒ) denote the space of all finite, finitely additive,ℒ-regular measures defined on the field of sets generated by ℒ . Generalizing the notion of an integral representation, in [5] Kirk and Crenshaw define a standard representation of A*, the Banach dual of A, in MR(ℒ) to be a linear map I of A* into MR(ℒ) with the property that if 0 ≦ ϕ ∈ A*, then

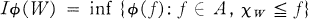
for every W in ℒ. The space MR(ℒ) is said to represent A* if there exists a (unique) standard representation I of A* onto MR(ℒ) which is a Banach lattice isomorphism.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 33 , Issue 2 , 01 April 1981 , pp. 372 - 380
Copyright
Copyright © Canadian Mathematical Society 1981

References

1. Brooks, R. M., On Wallman compactifications, Fund. Math. GO (1967), 157-173.Google Scholar
2. Dunford, N. and Schwartz, J. T., Linear operators, Part I (Interscience, New York, 1958).Google Scholar
3. Frink, O., Compactifications and seminormal spaces, Amer. J. Math. 86 (1964), 602–607.Google Scholar
4. Hegde, S., A characterization of z-separating algebras, Proc. Amer. Math. Soc. 73 (1979), 40–44.Google Scholar
5. Kirk, R. B. and Crenshaw, J. A., A generalized topological measure theory, Trans. Amer. Math. Soc. 207 (1975), 189–217.Google Scholar
6. Sultan, A., Measure, compactification and representation, Can. J. Math. 30 (1978), 54–65.Google Scholar
7. Varadarajan, V. S., Measures on topological spaces, Amer. Math. Soc. Transi. 48 (1965), 161–228.Google Scholar