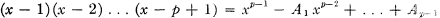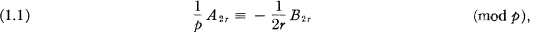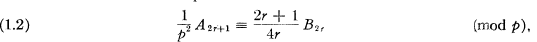Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Howard, F. T.
1990.
Extensions of Congruences of Glaisher and Nielsen Concerning Stirling Numbers.
The Fibonacci Quarterly,
Vol. 28,
Issue. 4,
p.
355.
Guy, Richard K.
1993.
A Quarter Century ofMonthlyUnsolved Problems, 1969–1993.
The American Mathematical Monthly,
Vol. 100,
Issue. 10,
p.
945.
Santmyer, Joseph M.
1997.
A stirling like sequence of rational numbers.
Discrete Mathematics,
Vol. 171,
Issue. 1-3,
p.
229.
Sun, Zhi-Hong
2000.
Congruences concerning Bernoulli numbers and Bernoulli polynomials.
Discrete Applied Mathematics,
Vol. 105,
Issue. 1-3,
p.
193.
Nyblom, M.A.
2002.
Congruences for the Coefficients within a Generalized Factorial Polynomial.
Rocky Mountain Journal of Mathematics,
Vol. 32,
Issue. 1,
Aicardi, Francesca
2008.
Recursive method for building Arnold’s triangle.
Functional Analysis and Other Mathematics,
Vol. 2,
Issue. 1,
p.
1.
MEŠTROVIĆ, ROMEO
2012.
AN EXTENSION OF SURY’S IDENTITY AND RELATED CONGRUENCES.
Bulletin of the Australian Mathematical Society,
Vol. 85,
Issue. 3,
p.
482.
HESSAMI PILEHROOD, KH.
HESSAMI PILEHROOD, T.
and
TAURASO, R.
2012.
CONGRUENCES CONCERNING JACOBI POLYNOMIALS AND APÉRY-LIKE FORMULAE.
International Journal of Number Theory,
Vol. 08,
Issue. 07,
p.
1789.
Sun, Zhi-Wei
2014.
p-adic congruences motivated by series.
Journal of Number Theory,
Vol. 134,
Issue. ,
p.
181.
MešTrović, Romeo
2017.
A Morley-Like Congruence Arising From Morley's Congruence.
Mathematics Magazine,
Vol. 90,
Issue. 2,
p.
142.
Mao, Guo-Shuai
and
Wang, Jie
2019.
On some congruences involving Domb numbers and harmonic numbers.
International Journal of Number Theory,
Vol. 15,
Issue. 10,
p.
2179.
Mao, Guo-Shuai
2023.
Proof of some congruence conjectures of Z.-H. Sun involving Apéry-like numbers.
Journal of Difference Equations and Applications,
Vol. 29,
Issue. 5,
p.
592.
Boumahdi, Rachid
Mihoubi, Miloud
and
Khaldi, Laala
2023.
On certain congruences involving central binomial coefficients.
Mathematica Montisnigri,
Vol. 58,
Issue. ,
p.
32.
Mao, Guo-Shuai
and
Zhang, Hao
2024.
On some super-congruences for the coefficients of analytic solutions of certain differential equations.
Indian Journal of Pure and Applied Mathematics,
Mechacha, Mohammed
2024.
Congruences involving quadrinomial coefficients.
Indian Journal of Pure and Applied Mathematics,