Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Mathieu, Martin
1988.
Elementary operators on prime C*-algebras II.
Glasgow Mathematical Journal,
Vol. 30,
Issue. 3,
p.
275.
Scholz, Erasmus
1993.
Completely continuous elementary operators on +( ).
Reports on Mathematical Physics,
Vol. 32,
Issue. 3,
p.
293.
Magajna, Bojan
1993.
A transitivity theorem for algebras of elementary operators.
Proceedings of the American Mathematical Society,
Vol. 118,
Issue. 1,
p.
119.
Coates, Keith J.
1994.
Algebraic Methods in Operator Theory.
p.
49.
Coates, Keith
1996.
Elementary operators and invariant subalgebras.
Proceedings of the American Mathematical Society,
Vol. 124,
Issue. 11,
p.
3437.
Saksman, Eero
and
Tylli, Hans-Olav
1998.
Rigidity of commutators and elementary operators on Calkin algebras.
Israel Journal of Mathematics,
Vol. 108,
Issue. 1,
p.
217.
Saksman, Eero
and
Tylli, Hans-Olav
1999.
The Apostol–Fialkow Formula for Elementary Operators on Banach Spaces.
Journal of Functional Analysis,
Vol. 161,
Issue. 1,
p.
1.
Mathieu, Martin
2001.
Recent Progress in Functional Analysis, Proceedings of the International Functional Analysis Meeting on the Occasion of the 70th Birthday of Professor Manuel Valdivia.
Vol. 189,
Issue. ,
p.
363.
Said, Bouali
and
Youssef, Bouhafsi
2010.
A remark on the range of elementary operators.
Czechoslovak Mathematical Journal,
Vol. 60,
Issue. 4,
p.
1065.
ECH-CHAD, Mohamed
2017.
Ranges and kernels of derivations.
TURKISH JOURNAL OF MATHEMATICS,
Vol. 41,
Issue. ,
p.
508.
Bouali, S.
Ech-Chad, M.
Bouhafsi, Y.
and
Missouri, M.
2018.
Intersections of Commutants with Closures of Derivation Ranges.
Analysis Mathematica,
Vol. 44,
Issue. 4,
p.
409.
Han, Yazhou
Sun, Ruifeng
and
Zhao, Xingpeng
2025.
On norm inequalities related to elementary operators in noncommutative fully symmetric spaces.
Positivity,
Vol. 29,
Issue. 1,
 and
and  denote complex Banach algebras and let
denote complex Banach algebras and let 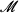 b e a left Banach
b e a left Banach  module and a right Banach
module and a right Banach  -module. If
-module. If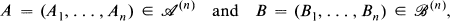
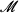 , by
, by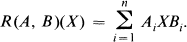
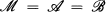 , elementary operators were introduced by Lumer and Rosenblum [19], who studied their spectral properties. In this setting many authors subsequently studied spectral, algebraic, metric, and structural properties of elementary operators, with particular attention devoted to the inner derivations δa (δa(x) = ax – xa) [25], generalized derivations τ(a, b) (τ(a, b)(x) = ax – xb) [9, 10], and elementary multiplications S(a, b) (S(a, b)(x) = axb), including left and right multiplications La and Rb [11].
, elementary operators were introduced by Lumer and Rosenblum [19], who studied their spectral properties. In this setting many authors subsequently studied spectral, algebraic, metric, and structural properties of elementary operators, with particular attention devoted to the inner derivations δa (δa(x) = ax – xa) [25], generalized derivations τ(a, b) (τ(a, b)(x) = ax – xb) [9, 10], and elementary multiplications S(a, b) (S(a, b)(x) = axb), including left and right multiplications La and Rb [11].