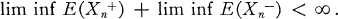Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-26T17:35:19.993Z Has data issue: false hasContentIssue false
  • English
  • Français

Strong Convergence of Pramarts in Banach Spaces

Published online by Cambridge University Press:  20 November 2018

Leo Egghe
Leo Egghe*
Affiliation:
L. U. C-Universitaire Campus, Diepenbeek, Belgium
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let E be a Banach space and 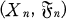be an adapted sequence on the probability space 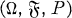We denote by T the set of all bounded stopping times with respect to 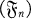. 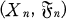is called a pramart if

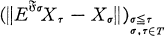
converges to zero in probability, uniformly in τ ≧ σ. The notion of pramart was introduced in [6]. A good property is the optional sampling property (see Theorem 2.4 in [6]). Furthermore the class of pramarts intersects the class of amarts, and every amart is a pramart if and only if dim E < ∞ ([2], see also [4]). Pramarts behave indeed quite differently than amarts. Although the class of pramarts is large, they have good convergence properties as is seen in the next two results of Millet-Sucheston, [6], [7].

THEOREM 1.1. Let 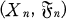be a real-valued pramart of class (d), i.e.,

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 33 , Issue 2 , 01 April 1981 , pp. 357 - 361
Copyright
Copyright © Canadian Mathematical Society 1981

References

1. Brunei, A. and Sucheston, L., On B-convex Banach spaces, Math. Systems Theory 7 (1973), 294–299.Google Scholar
2. Edgar, G. A. and Sucheston, L., Martingales in the limit and amarts, Proc. AMS 67 (1977), 315–320.Google Scholar
3. Edgar, G. A. and Sucheston, L., Amarts, a class of asymptotic martingales ﹛discrete parameter), J. Multivariate Analysis 6 (1976), 193–221.Google Scholar
4. Egghe, L., Weak and strong convergence of amarts in Fréchet spaces, To appear in J. Multivariate Analysis.Google Scholar
5. Meyer, P. A., Probability and potentials (Blaisdell Publishing Company, Waltham-Toronto-London, 1966).Google Scholar
6. Millet, A. and Sucheston, L., Convergence of classes of amarts, indexed by directed sets, Can. J. Math. 31 (1979), 1033–1046.Google Scholar
7. Millet, A. and Sucheston, L., Characterizations of Vitali conditions with overlap in terms of convergence of classes of amarts, Can. J. Math. 82 (1980), 86–125.Google Scholar