Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-24T00:44:40.635Z Has data issue: false hasContentIssue false
  • English
  • Français

A Skolem–Mahler–Lech Theorem for Iterated Automorphisms of K–algebras

Published online by Cambridge University Press:  20 November 2018

Jason P. Bell  and
Jeffrey C. Lagarias
Jason P. Bell
Affiliation:
Department of Pure Mathematics, University of Waterloo, Waterloo, ON, N2L 3G1. e-mail: [email protected]
Jeffrey C. Lagarias
Affiliation:
Department of Mathematics, University of Michigan, Ann Arbor, MI 48109-1043, USA. email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper we prove a commutative algebraic extension of a generalized Skolem–Mahler– Lech theorem. Let 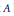$A$ be a finitely generated commutative 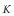$K$–algebra over a field of characteristic 0, and let 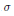$\sigma$ be a 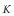$K$–algebra automorphism of 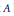$A$. Given ideals 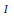$I$ and 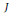$J$ of 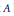$A$, we show that the set 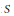$S$ of integers $m$ such that 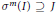${{\sigma }^{m}}(I)\,\supseteq \,J$ is a finite union of complete doubly infinite arithmetic progressions in $m$, up to the addition of a finite set. Alternatively, this result states that for an affine scheme 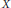$X$ of finite type over 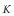$K$, an automorphism 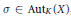$\sigma \,\in \,\text{Au}{{\text{t}}_{k}}(X)$, and $Y$ and 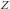$Z$ any two closed subschemes of 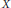$X$, the set of integers $m$ with 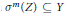${{\sigma }^{m}}(Z)\,\subseteq \,Y$ is as above. We present examples showing that this result may fail to hold if the affine scheme 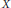$X$ is not of finite type, or if 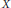$X$ is of finite type but the field 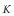$K$ has positive characteristic.

Keywords

11D4514R1011Y5511D88automorphismsendomorphismsaffine spacecommutative algebrasSkolem–Mahler–Lech theorem
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 67 , Issue 2 , April 2015 , pp. 286 - 314
Copyright
Copyright © Canadian Mathematical Society 2015

References

[1] Allouche, J.-P. and Shallit, J., Automatic sequencets. Theory, applications, generalizations. Cambridge University Press, Cambridge, 2003 Google Scholar
[2] Amitsur, A. S., Algebras over infinite fields. Proc. Amer. Math. Soc. 7(1956), 35– 48.http://dx.doi.org/10.1090/S0002-9939-1956-0075933-2 Google Scholar
[3] Atiyah, M. F. and Macdonald, I. G., Introduction to commutative algebra. Addison-Wesley Publishing Co., Reading, Mass.-London-Don Mills, ON, 1969.Google Scholar
[4] Bell, J. P., A generalised Skolem– Mahler– Lech theorem for affine varieties. J. London Math. Soc. 73(2006), no. 2, 367– 379.http://dx.doi.org/10.1112/S002461070602268X Google Scholar
[5] Bell, J. P., Corrigendum to: “A generalised Skolem– Mahler– Lech theorem for affine varieties”. J. London Math. Soc. 78(2008), 267– 272. http://dx.doi.org/10.1112/jlms/jdn012 Google Scholar
[6] Bell, J. P., Ghioca, D., and Tucker, T. J., The dynamical Mordell-Lang problem for étale maps. Amer. J. Math. 132(2010), no. 6, 1655– 1675.Google Scholar
[7] Benedetto, R. L., Ghioca, D., Kurlberg, P., and Tucker, T. J., A case of the dynamical Mordell– Lang conjecture. Math. Ann. 352(2012), no. 1, 1– 26.http://dx.doi.org/10.1007/s00208-010-0621-4 Google Scholar
[8] Bézivin, J.-P., Une généralisation du théorème de Skolem– Mahler– Lech. Quart. J. Math. Oxford Ser. (2) 40(1989), no. 158, 133– 138.http://dx.doi.org/10.1093/qmath/40.2.133 Google Scholar
[9] Cahen, P. J. and Chabert, J.-L., Integer– valued polynomials. Mathematical Surveys and Monographs, 48, American Mathematical Society, Providence, RI, 1997.Google Scholar
[10] Cassels, J.W. S., Local fields. London Mathematical Society Student Texts, 3, Cambridge University Press, Cambridge, 1986.Google Scholar
[11] Denis, L., Géométrie et suites récurrentes. Bull. Soc. Math. France 122(1994), no. 1, 13– 27.Google Scholar
[12] Derksen, H., A Skolem– Mahler– Lech theorem in positive characteristic and finite automata. Invent. Math. 168(2007), no. 1, 175– 224.http://dx.doi.org/10.1007/s00222-006-0031-0 Google Scholar
[13] Eisenbud, D., Commutative algebra. With a view towards algebraic geometry. Graduate Texts in Mathematics, 150, Springer– Verlag, New York, 1995.Google Scholar
[14] Everest, G., van der Poorten, A., Shparlinski, I., and Ward, T., Recurrence sequences. Mathematical Surveys and Monographs, 104, American Mathematical Society, Providence, RI, 2003.Google Scholar
[15] Farkas., D. R. Recurrent behavior in rings. J. Algebra 108(1987), no. 1, 127– 138.http://dx.doi.org/10.1016/0021-8693(87)90126-8 Google Scholar
[16] Ghioca, D. and Tucker, T. J., Periodic points, linearizing maps, and the dynamical Mordell– Lang problem. J. Number Theory 129(2009), no. 6, 1392– 1403.http://dx.doi.org/10.1016/j.jnt.2008.09.014 Google Scholar
[17] Ghioca, D., Tucker, T. J., and M. E., Zieve, Intersections of polynomial orbits, and a dynamical Mordell– Lang conjecture. Invent. Math. 171(2008), no. 2, 463– 483.http://dx.doi.org/10.1007/s00222-007-0087-5 Google Scholar
[18] Hansel, G., Une démonstration simple du théorème de Skolem– Mahler– Lech. Theoret. Comput. Sci. 43(1986), no. 1, 91– 98. http://dx.doi.org/10.1016/0304-3975(86)90168-4 Google Scholar
[19] Hartshorne, R., Algebraic geometry. Graduate Texts in Mathematics, 52, Springer– Verlag, New York, 1977.Google Scholar
[20] Lech, C., A note on recurring series. Ark. Mat. 2(1953), 417– 421.http://dx.doi.org/10.1007/BF02590997 Google Scholar
[21] Mahler, K, Eine arithmetische Eigenshaft der Taylor– Koeffizienten rationaler Funktionen, Proc. Kon. Nederlandsche Akad. v.Wetenschappen, 38(1935), 50– 60.Google Scholar
[22] Mahler, K, On the Taylor coefficients of rational functions. Proc. Cambridge Philos. Soc. 52(1956), 39– 48.http://dx.doi.org/10.1017/S0305004100030966 Google Scholar
[23] Mahler, K, Addendum to the paper “On the Taylor coefficients of rational functions”. Proc. Cambridge Philos. Soc. 53(1957), 544. http://dx.doi.org/10.1017/S0305004100032552 Google Scholar
[24] Mahler, K, p– adic numbers and their functions. Second ed., Cambridge Tracts in Mathematics, 76, Cambridge University Press, Cambridge, New York, 1981.Google Scholar
[25] Poonen, B., p– adic interpolation of iterates.. http://arxiv:1307.5887 Google Scholar
[26] Sierra, S. J., Geometric idealizer rings. Trans. Amer. Math. Soc. 363(2011), no. 1, 457– 500.http://dx.doi.org/10.1090/S0002-9947-2010-05110-4 Google Scholar
[27] Skolem, T., Einige Sätze Über gewisse Reihenentwicklungen und exponentiale Beziehungen mit Anwendung auf Diophantische Gleichungen.Skrifter Norske Vidensk. Acad. Oslo, Mat. Naturv. Klasse (1933), no. 6.Google Scholar
[28] Skolem, T., Ein Verfahren zur Behandlung gewisser exponentialer Gleichungen und diophantischer Gleichungen. C. r. 8 congr. scand. à Stockholm (1934), 163– 188.Google Scholar
[29] Srinivas, V., On the embedding dimension of an affine variety. Math. Ann. 289(1991), no. 1, 125– 132.http://dx.doi.org/10.1007/BF01446563 Google Scholar
[30] Strassman, R., Über den Wertevorrat von Potenzreihen im Gebiet der p-adischen Zahlen. J. Reine Angew. Math. 159(1928), 13– 28; 65– 66.Google Scholar
[31] van der Poorten, A. J., Some facts that should be better known; especially about rational functions. In: Number theory and applications (Banff, AB, 1988), NATO Adv. Sci. Inst. Ser. C Math. Phys. Sci., 265, Kluwer Academic Publishers, Dordrecht, 1989, pp. 497– 528.Google Scholar
[32] van der Poorten, A. J. and Tijdeman, R., On common zeros of exponential polynomials. Enseignement Math. (2) 21(1975), no. 1, 57– 67.Google Scholar
[33] Zhang, S.-W., Distributions in algebraic dynamics. Surv. Differ. Geom. 10(2006), 381– 430.Google Scholar