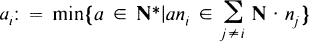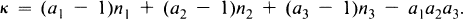Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Patil, Dilip P.
and
Singh, Balwant
1990.
Generators for the derivation modules and the relation ideals of certain curves.
Manuscripta Mathematica,
Vol. 68,
Issue. 1,
p.
327.
Molinelli, Silvia
and
Tamone, Grazia
1993.
On the derivations of semigroup rings.
Rendiconti del Circolo Matematico di Palermo,
Vol. 42,
Issue. 3,
p.
459.
Patil, D.P.
and
Sengupta, I.
1999.
Minimal set of generators for the derivation module of certain monomial curves.
Communications in Algebra,
Vol. 27,
Issue. 11,
p.
5619.
Fel, Leonid G.
2007.
Frobenius problem for semigroups $\mathsf{S}(d_{1},d_{2},d_{3})$.
Functional Analysis and Other Mathematics,
Vol. 1,
Issue. 2,
p.
119.
Fel, Leonid G.
2008.
Analytic representations in the three-dimensional Frobenius problem.
Functional Analysis and Other Mathematics,
Vol. 2,
Issue. 1,
p.
27.
Patil, D.P.
and
Tamone, G.
2011.
CM defect and Hilbert functions of monomial curves.
Journal of Pure and Applied Algebra,
Vol. 215,
Issue. 7,
p.
1539.
Katsabekis, Anargyros
2019.
Complete Intersection Monomial Curves and the Cohen—Macaulayness of Their Tangent Cones.
Algebra Colloquium,
Vol. 26,
Issue. 04,
p.
629.
Saha, Joydip
and
Sengupta, Indranath
2022.
Derivation modules for sum and gluing.
Proceedings - Mathematical Sciences,
Vol. 132,
Issue. 1,
Sengupta, Indranath
2022.
Affine monomial curves.
Proceedings - Mathematical Sciences,
Vol. 132,
Issue. 1,
Pandit, Sudip
Saha, Joydip
and
Sengupta, Indranath
2023.
Numerical semigroups with unique Apéry expansions.
Journal of Algebra and Its Applications,
Vol. 22,
Issue. 09,
Bhardwaj, Om Prakash
and
Sengupta, Indranath
2024.
DERIVATION MODULE AND THE HILBERT–KUNZ MULTIPLICITY OF THE COORDINATE RING OF A PROJECTIVE MONOMIAL CURVE.
Rocky Mountain Journal of Mathematics,
Vol. 54,
Issue. 3,
Patra, Amit
2024.
Number of generators of derivation modules of some one-dimensional local domain.
Communications in Algebra,
Vol. 52,
Issue. 9,
p.
3711.
Laface, Antonio
and
Ugaglia, Luca
2025.
On Blowing Up Minimal Toric Surfaces.
International Mathematics Research Notices,
Vol. 2025,
Issue. 6,