Published online by Cambridge University Press: 20 November 2018
We consider minimal left ideals L of the universal semigroup compactification 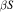 of a topological semigroup S. We show that the enveloping semigroup of L is homeomorphically isomorphic to
of a topological semigroup S. We show that the enveloping semigroup of L is homeomorphically isomorphic to 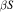 if and only if given q ≠ r in
if and only if given q ≠ r in 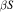 , there is some p in the smallest ideal of
, there is some p in the smallest ideal of 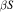 with qp ≠ rp. We derive several conditions, some involving minimal flows, which are equivalent to the ability to separate q and r in this fashion, and then specialize to the case that S =
with qp ≠ rp. We derive several conditions, some involving minimal flows, which are equivalent to the ability to separate q and r in this fashion, and then specialize to the case that S =  , and the compactification is
, and the compactification is 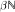 . Included is the statement that some set A whose characteristic function is uniformly recurrent has
. Included is the statement that some set A whose characteristic function is uniformly recurrent has 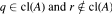 .
.
 , Semigroup Forum
44(1992), 107–111.Google Scholar
, Semigroup Forum
44(1992), 107–111.Google Scholar , Semigroup Forum
25(1982), 291–310.Google Scholar
, Semigroup Forum
25(1982), 291–310.Google Scholar on the torus group, Semigroup Forum
43(1991), 202–217.Google Scholar
on the torus group, Semigroup Forum
43(1991), 202–217.Google Scholar