Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-26T12:36:31.022Z Has data issue: false hasContentIssue false
  • English
  • Français

Scattering Theory And Spectral Representations For General Wave Equations With Short Range Perturbations

Published online by Cambridge University Press:  20 November 2018

Kazuhiro Yamamoto
Kazuhiro Yamamoto*
Affiliation:
Department of Mathematics, Nagoya Institute of Technology, Nagoya, Gokiso-cho, 466 Japan
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper we shall develop the scattering theory introduced by Lax and Phillips [5] for the following general wave equation; 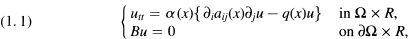where Ω is an exterior domain Rn(n ≥ 3) with the smooth boundary δΩ and B is either a Dirichlet boundary condition or of the form Bu = Vi(x)aij(x)δju+σ(x)u with the unit outer normal vector v(x) = (v1 , … , vn) at x ∈ δ Ω. The precise assumptions on α(x), aij(x),q(x), σ(x) are denoted below. If Ω is an inhomogeneous medium with the density ρ (x), the propagation of waves is described by (1.1) with a(x) = a(x)2 ρ(x), aij(x) = ρ-l(x) δij and q(x) = 0 with the velocity a(x).

Keywords

35L35P
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 43 , Issue 2 , 01 April 1991 , pp. 435 - 448
Copyright
Copyright © Canadian Mathematical Society 1991

References

1. Agmon, S., Elliptic boundary value problems. D. Van Nostrand, New York, 1965.Google Scholar
2. Hörmander, L., Linear partial differential operators. Springer-Verlag, New York, 1963.Google Scholar
3. Ikebe, T., Spectral representation for Schrödinger operators with long-range potentials, J. Functional Anal. 20(1975), 158–176.Google Scholar
4. Ikebe, T., Spectral representation for Schrödinger operators with long-range potentials II, perturbation by short-range potentials, Publ. RIMS, Kyoto Univ. 11(1976), 551–558.Google Scholar
5. Lax, P.D. and Phillips, R.S., Scattering theory. Academic Press, New York, 1967.Google Scholar
6. Lax, P.D. Scattering theory for the acoustic equations in an even number of space dimensions, Indiana Univ. Math. J. 22(1972), 101–134.Google Scholar
7. Lax, P.D. The acoustic equation with an indefinite energy form and the Schrodinger equation, J. Functional Analysis 1(1967), 37–87.Google Scholar
8. Mochizuki, K., Spectral and scattering theory for second order elliptic differential operators in an exterior domain. Lecture Note Univ. Utah, Winter and Spring, 1972.Google Scholar
9. Mochizuki, K., Scattering theory for the wave equation. Kinokuniya Shoten, Tokyo, 1984 (in Japanese).Google Scholar
10. Mochizuki, K., Growth properties of solutions of second order elliptic differential equations, J. Math. Kyoto Univ. 16(1976), 351–373.Google Scholar
11. Phillips, R.S., Scattering theory for the wave equation with a short range perturbation, Indiana Univ. Math. J. 31(1982), 609–639.Google Scholar
12. Reed, M. and Simon, B., Methods of modern mathematical physics, Vol. III. Academic Press, New York, 1979.Google Scholar
13. Shenk, N.A., Eigen function expansions and scattering theory for the wave equation in an exterior region, Arch. Rat. Mech. Anal. 21(1966), 120–150.Google Scholar
14. Thoe, D., Spectral theory for the wave equation with a potential term, Arch. Rat. Mech. Anal. 22(1966), 364–406.Google Scholar
15. Yamamoto, K., Existence of outgoing solutions for perturbations of —A and applications to scattering theory, (to appear).Google Scholar
16. Yosida, K., Functional Analaysis. Springer-Verlag, New York, 1965.Google Scholar