Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-24T02:29:51.142Z Has data issue: false hasContentIssue false
  • English
  • Français

Résonances près de seuils d'opérateurs magnétiques de Pauli et de Dirac

Published online by Cambridge University Press:  20 November 2018

Diomba Sambou
Diomba Sambou*
Affiliation:
Univ. BordeauxInstitut de Mathématiques de Bordeaux, UMR 5251, Université de Bordeaux I, F-3340 Talence, France, courriel: [email protected]
Rights & Permissions [Opens in a new window]

Résumé

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

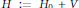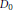Nous considérons les perturbations $H\,:=\,{{H}_{0}}\,+\,V$ et $D\,:=\,{{D}_{0}}\,+\,V$ des Hamiltoniens libres ${{H}_{0}}$ de Pauli et ${{D}_{0}}$ de Dirac en dimension 3 avec champ magnétique non constant, $V$ étant un potentiel électrique qui décroıt super-exponentiellement dans la direction du champ magnétique. Nous montrons que dans des espaces de Banach appropriés, les résolvantes de 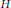$H$ et 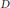$D$ définies sur le demi-plan supérieur admettent des prolongements méromorphes. Nous définissons les résonances de 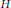$H$ et 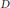$D$ comme étant les pôles de ces extensions méromorphes. D’une part, nous étudions la répartition des résonances de 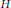$H$ prés de l’origine 0 et d’autre part, celle des résonances de 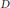$D$ près de $\pm m$ où m est la masse d’une particule. Dans les deux cas, nous obtenons d’abord des majorations du nombre de résonances dans de petits domaines au voisinage de 0 et $\pm m$. Sous des hypothèses supplémentaires, nous obtenons des développements asymptotiques du nombre de résonances qui entraınent leur accumulation près des seuils 0 et $\pm m$. En particulier, pour une perturbation $V$ de signe défini, nous obtenons des informations sur la répartition des valeurs propres de $H$ et $D$ près de 0 et $\pm m$ respectivement.

Keywords

35B3435P25opérateurs magnétiques de Pauli et de Diracrésonances.
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 65 , Issue 5 , 01 October 2013 , pp. 1095 - 1124
Copyright
Copyright © Canadian Mathematical Society 2013

References

Références

[1] Adam, C., Muratori, B., et Nash, C., Zero modes of the Dirac operator in the three dimensions. Phys. Rev. D 60(1999), 125001–1–125001–8. http://dx.doi.org/10.1103/PhysRevD.60.125001 Google Scholar
[2] Adam, C., Degeneracy of zero modes of the Dirac operator in the three dimension. Phys. Lett. B 485(2000), 314–318. http://dx.doi.org/10.1016/S0370-2693(00)00701-2 Google Scholar
[3] Adam, C., Multiple zero modes of the Dirac operator in the three dimensions. Phys. Rev. D 62(2000), 085026-1–085026-9. http://dx.doi.org/10.1103/PhysRevD.62.085026 Google Scholar
[4] Avron, J., Herbst, I., et Simon, B., Schrödinger operators with magnetic fields. I. General interactions. Duke Math. J. 45(1978), 847–883. http://dx.doi.org/10.1215/S0012-7094-78-04540-4 Google Scholar
[5] Balinsky, A. A. etW. Evans, D., On the zero modes of the Weyl–Dirac operators and their multiplicity. Bull. London Math. Soc. 34(2002), 236–242. http://dx.doi.org/10.1112/S0024609301008736 Google Scholar
[6] Bony, J. F., Bruneau, V., et Raikov, G., Resonances and Spectral Shift Function near the Landau levels. Ann. Inst. Fourier 57(2007), 629–671. http://dx.doi.org/10.5802/aif.2270 Google Scholar
[7] Bony, J. F., Counting function of characteristic values and magnetic resonances. Preprint, arxiv:arxiv.org/abs/1109.3985.Google Scholar
[8] Bruneau, V., Pushnitski, A., et Raikov, G., Spectral shift function in strong magnetic fields. Algebra in Analiz, 16(2004), 207–238.Google Scholar
[9] Boutet de Monvel, A. M. et Purice, R., On the theory of wave operators and scattering operators. Dokl. Akad. Nauk. S.S.S.R. 5(1962), 475–478.Google Scholar
[10] Boutet de Monvel, A. M., A distinguished self-adjoint extension for the Dirac operator with strong local singularities and arbitrary behaviour at infinity. Rep. Math. Phys. 34(1994), 351–360. http://dx.doi.org/10.1016/0034-4877(94)90007-8 Google Scholar
[11] Chernoff, Paul R., Schrödinger and Dirac operators with singular potentials and hyperbolic equations. Pacific J. Math. 72(1977), 361–382. http://dx.doi.org/10.2140/pjm.1977.72.361 Google Scholar
[12] Dimassi, M. et Sjöstrand, J., Spectral Asymptotics in the Semi-classical Limit. London Math. Soc. Lecture Note Ser. 268, Cambridge, Cambridge University Press, 1999.Google Scholar
[13] Fernandez, C. et Raikov, G. D., On the singularities of the magnetic spectral shift function at the landau levels. Ann. Henri Poincaré 5(2004), 381–403. http://dx.doi.org/10.1007/s00023-004-0173-9 Google Scholar
[14] Georgescu, V. et Mantoiu, M., On the spectral theory of singular Dirac type Hamiltonians. J. Operator Theory 46(2001), 289–321.Google Scholar
[15] Gohberg, I. et Sigal, E. I., An operator generalization of the logarithmic residue theorem and Rouché’s theorem. Mat. Sb. (N.S.) 84(1971), 607–629.Google Scholar
[16] Gohberg, I. et Leiterer, J., Holomorphic Operator Functions of One variable and Applications. Operator Theory Advances and Applications 192, Birkhaöser, Basel. Boston. Berlin, 2009.Google Scholar
[17] Hall, B. C., Holomorphic methods in analysis and mathematical physics. Dans: First Summer School in Analysis and Mathematical Physics (Cuernavaca Morelos, 1998), Contemp. Math. 260, American Mathematical Society, Providence, RI, 2000, 1–59.Google Scholar
[18] Helffer, B., Nourrigat, J. et Wang, X. P., Sur le spectre de l’équation de Dirac (dans R2 ou R3) avec champ magnétique. Ann. Sci. Ecole Norm. Sup. 22(1989), 515–533.Google Scholar
[19] Khochman, A., Resonances and spectral shift function for the semi-classical Dirac operator. Rev. Math. Phys. 19(2007), 1071–1115. http://dx.doi.org/10.1142/S0129055X0700319X Google Scholar
[20] Koplienko, L. S., Trace formula for non trace-class perturbations. Sibirsk. Mat. Zh. 25(1984), 62–71; (English) Siberian Math. J. 25(1984), 735–743.Google Scholar
[21] Loss, M. et Yau, H. T., Stability of Coulomb systems with magnetic fields. III. Zero energy bound states of the Pauli operators. Commun. Math. Phys. 104(1986), 283–290. http://dx.doi.org/10.1007/BF01211595 Google Scholar
[22] Raikov, G. D., Spectral asymptotics for the perturbed 2D Pauli Operator with oscillating magnetic Fields. I. Non-zero mean value of the magnetic field. Markov Process. Related Fields 9(2003), 775–794.Google Scholar
[23] Raikov, G. D., Low Energy Asymptotics of the SSF for Pauli Operators with Nonconstant Fields. Publ. Res. Inst. Math. Sci. Kyoto Univ. 46(2010), 565–590. http://dx.doi.org/10.2977/PRIMS/18 Google Scholar
[24] Raikov, G. D. et Warzel, S., Quasi-classical versus non-classical spectral asymptotics for magnetic Schrödinger operators with decreasing electric potentials. Rev. Math. Phys. 14(2002), 1051–1072. http://dx.doi.org/10.1142/S0129055X02001491 Google Scholar
[25] Reed, M. et Simon, B., Methods of Modern Mathematical Physics III. Scattering Theory. Academic Press, Inc., New York–London, 1979.Google Scholar
[26] Richard, S. et Tiedra de Aldecoa, R., On the spectrum of magnetic Dirac operators with Coulomb-type perturbations. J. Funct. Anal. 250(2007), 625–641. http://dx.doi.org/10.1016/j.jfa.2007.04.016 Google Scholar
[27] Saito, Y. et Umeda, T., The asymptotic limits of zero modes of massless Dirac operators. Lett. Math. Phys. 83(2008), 97–106. http://dx.doi.org/10.1007/s11005-007-0207-6 Google Scholar
[28] Saito, Y., The zero modes and zero resonances of massless Dirac operators. Hokkaido Math. J. 37(2008), 363–388.Google Scholar
[29] Saito, Y., Eigenfunctions at the threshold energies of magnetic Dirac operators. Rev. Math. Phys. 23(2011), 155–178. http://dx.doi.org/10.1142/S0129055X11004254 Google Scholar
[30] Simon, B., Trace ideals and their applications. London Math. Soc. Lecture Note Ser. 35, Cambridge, Cambridge University Press, 1979.Google Scholar
[31] Sjostrand, J., Lectures on resonances. Preprint, www.math.polytechnique.fr/$nthicksim$sjostrand/. Google Scholar
[32] Sjostrand, J., Weyl law for semi-classical resonances with randomly perturbed potentials. Preprint, arxiv.org/abs/1111.3549.Google Scholar
[33] Tiedra de Aldecoa, R., Asymptotics near ±m of the spectral shift function for Dirac operators with non-constant magnetic fields. Comm. Partial Differential Equations 36(2011), 10–41. http://dx.doi.org/10.1080/03605301003758369 Google Scholar
[34] Thaller, B., The Dirac equation. Springer-Verlag, Berlin, 1992.Google Scholar
[35] Yafaev, D. R., Mathematical scattering theory. General theory. Trans. Math. Monogr. 105, American Mathematical Society, Providence, RI, 1992.Google Scholar