Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-26T06:05:37.142Z Has data issue: false hasContentIssue false
  • English
  • Français

Resolution D'Equations Associees a un Systeme de Tchebycheff

Published online by Cambridge University Press:  20 November 2018

Serge Dubuc  and
Jean Savoie
Serge Dubuc
Affiliation:
Université de Montréal, Montréal, Québec
Jean Savoie
Affiliation:
Université de Montréal, Montréal, Québec
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Soit {f1(x),f2(x), …,fm(x)} un système de Tchebycheff de dimension m, c'est-à-dire les fonctions fj(x) sont des fonctions réelles continues définies sur un intervalle ouvert (a, b) et toute fonction de la forme 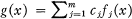admet au plus m — 1 racines dans (a, b) lorsqu'au moins un des coefficients Cj diffère de 0. Notre point de départ est un théorème dû à Krein [3]. On se limite au cas où la dimension m est paire, m = 2n. Ce théorème dit que la totalité Ω des points 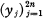de R2n qui se représentent ainsi 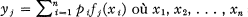sont n points distincts de (a, b) et où pi > 0, 1 ≦ i≦n, forme effectivement un ouvert convexe de R2n. Les systèmes d'équations que nous voulons traiter sont justement les équations:

I

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 34 , Issue 3 , 01 June 1982 , pp. 593 - 620
Copyright
Copyright © Canadian Mathematical Society 1982

References

Bibliographie

1. Bellman, R. E., Kalaba, R. E. et Lockett, J. A., Numerical inversion of the Laplace transform: applications to biology, economics and physics (American Elsevier, New York, 1966.Google Scholar
2. Karlin, S. et Studden, W., Tchebycheff systems: with application in analysis and statistics (Interscience, New York, 1966.Google Scholar
3. Krein, M. G., The ideas of P. L. Cebysev and A. A. Markov in the theory of integrals and their further development, Uspehi Mat. Nauk (N.S.) 6 (1951), (44), 3–120. (Amer. Math. Soc. Transi. 12 (1959), 1-121).Google Scholar
4. Krein, M. G. et Nudel'man, A. A., The Markov moment problem and extremal problems, Nauka, Moscou (1973). Traduction anglaise: American Mathematical Society (1977).Google Scholar
5. Kronrod, A. S., Nodes and weights of quadrature formulas (Consultants Bureau, New York, 1965.Google Scholar
6. Rutishauser, H., Der Quotienten-Differenzen Algorithmus (Birkhaùser, Verlag, Basel, 1957).Google Scholar
7. Savoie, J., Résolution numérique d'un système d'équations non linéaires déterminé par un système de Tchebycheff, Thèse de doctorat, Université de Montréal (1981).Google Scholar