Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-23T10:02:39.892Z Has data issue: false hasContentIssue false
  • English
  • Français

Representation of Banach Ideal Spaces and Factorization of Operators

Published online by Cambridge University Press:  20 November 2018

Evgenii I. Berezhnoĭ  and
Lech Maligranda
Evgenii I. Berezhnoĭ
Affiliation:
Department of Mathematics, Yaroslavl’ State University, Sovetskaya 14, 150 000 Yaroslavl’, Russia, email: [email protected]
Lech Maligranda
Affiliation:
Department of Mathematics, Luleå University of Technology, SE-971 87 Luleå, Sweden, email: [email protected] website: www.sm.luth.se/∽lech/
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

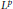Representation theorems are proved for Banach ideal spaces with the Fatou property which are built by the Calderón–Lozanovskiĭ construction. Factorization theorems for operators in spaces more general than the Lebesgue ${{L}^{p}}$ spaces are investigated. It is natural to extend the Gagliardo theorem on the Schur test and the Rubio de Francia theorem on factorization of the Muckenhoupt 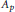${{A}_{p}}$ weights to reflexive Orlicz spaces. However, it turns out that for the scales far from 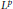${{L}^{p}}$-spaces this is impossible. For the concrete integral operators it is shown that factorization theorems and the Schur test in some reflexive Orlicz spaces are not valid. Representation theorems for the Calderón–Lozanovskiĭ construction are involved in the proofs.

Keywords

46E3046B4246B70Banach ideal spacesweighted spacesweight functionsCalderón–Lozanovskiĭ spacesOrlicz spacesrepresentation of spacesuniqueness problempositive linear operatorspositive sublinear operatorsSchur testfactorization of operatorsfactorization of weightscomplex interpolationmethodreal interpolation method.
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 57 , Issue 5 , 01 October 2005 , pp. 897 - 940
Copyright
Copyright © Canadian Mathematical Society 2005

References

[1] Asekritova, I. and Krugljak, N., On equivalence of K- and J-methods for (n + 1)-tuples of Banach spaces. Studia Math. 122(1997), 99–116.Google Scholar
[2] Bennett, C. and Sharpley, R., Interpolation of Operators. Academic Press, Boston 1988.Google Scholar
[3] Berezhnoĭ, E. I., Interpolation of positive operators in the spaces ϕ(X0, X1). In: Qualitative and Approximate Methods for the Investigation of Operator Equations, Yaroslav. Gos. Univ., Yaroslavl 1981, pp. 3–12 (Russian).Google Scholar
[4] Berezhnoĭ, E. I., Theorems on the representation of spaces and Schur's lemma. Dokl. Akad. Nauk 344(1995), 727–729; English Transl. in Doklady Math. 52(1995), 252–254.Google Scholar
[5] Berezhnoĭ, E. I., An inverse problem in the theory of the interpolation of operators. Mat. Zametki 59(1996), 323–333; English Transl. in Math. Notes 59(1996), 227–233.Google Scholar
[6] Berezhnoĭ, E. I. and Mastyło, M., On Calderón–Lozanovskiĭ construction. Bull. Polish Acad. Sci. Math. 37(1989), 23–33.Google Scholar
[7] Berezhnoĭ, E. I., The Lions problem for Gustavson–Peetre functor. Publ. Math. 34(1990), 175–180.Google Scholar
[8] Bergh, J. and Löfström, J., Interpolation Spaces. Springer, Berlin 1976.Google Scholar
[9] Bloom, S., Solving weighted norm inequalities using the Rubio de Francia algorithm. Proc. Amer. Math. Soc. 101(1987), 306–312.Google Scholar
[10] Brudnyĭ, Yu. A. and Krugljak, N. Ya., Interpolation Functors and Interpolation Spaces I. North-Holland, Amsterdam 1991.Google Scholar
[11] Calder ón, A. P., Intermediate spaces and interpolation, the complex method. Studia Math. 24(1964), 113–190.Google Scholar
[12] Christ, M., Weighted norm inequalities and Schur's lemma. Studia Math. 78(1984), 309–319.Google Scholar
[13] Coifman, R., Jones, P.W., and Rubio de Francia, J. L., Constructive decomposition of BMO functions and factorization of Ap weights. Proc. Amer.Math. Soc. 87(1983), 675–676.Google Scholar
[14] Cwikel, M., Nilsson, P. G. and Schechtman, G., Interpolation of Weighted Banach Lattices/ A Characterization of Relatively Decomposable Banach Lattices. Mem. Amer.Math. Soc. 787, 2003.Google Scholar
[15] Gagliardo, E., On integral transformations with positive kernel. Proc. Amer. Math. Soc. 16(1965), 429–434.Google Scholar
[16] Garcia-Cuerva, J. and Rubio de Francia, J., Weighted Norm Inequalities and Related Topics. North-Holland, Amsterdam, 1985.Google Scholar
[17] Hernández, E., Factorization and extrapolation of pairs of weights. Studia Math. 45(1989), 179–193.Google Scholar
[18] Hernández, E., Weighted inequalities through factorization. Publ. Math. 35(1991), 141–153.Google Scholar
[19] Janson, S., Nilsson, P., and Peetre, J., Notes on Wolff 's note on interpolation spaces. Proc. London Math. Soc. 48(1984), 283–299.Google Scholar
[20] Jawerth, G. B.,Weighted inequalities for maximal operators: linearization, localization and factorization. Amer. J. Math. 108(1986), 361–414.Google Scholar
[21] Jones, P., Factorization of Ap-weights. Ann. of Math. 111(1980), 511–530.Google Scholar
[22] Kami ńska, A., Maligranda, L., and Persson, L. E., Indices, convexity and concavity of Calderón–Lozanovskiĭ spaces. Math. Scand. 92(2003), 141–160.Google Scholar
[23] Korotkov, V. B., Integral Operators. Nauka Sibirsk. Otdel., Novosibirsk, 1983, (Russian).Google Scholar
[24] Krbec, M. and Pick, L., On imbeddings between weighted Orlicz spaces. Z. Anal. Anwendungen 10(1991), 107–117.Google Scholar
[25] Krein, S. G., Petunin, Yu. I., and Semenov, E. M., Interpolation of Linear Operators. Nauka, Moscow, 1978; Translations of Mathematical Monographs 54, American Mathematical Society, Providence, RI, 1982.Google Scholar
[26] Krugljak, N. and Maligranda, L., Calderón–Lozanovskiĭ construction on weighted Banach function lattices. J. Math. Anal. Appl. 288(2003), 744–757.Google Scholar
[27] Lindenstrauss, J. and Tzafriri, L., Classical Banach Spaces, II. Function Spaces. Springer-Verlag, Berlin, New York, 1979.Google Scholar
[28] Lions, J. L. and Magenes, E., Problèmes aux Limites Non Homogènes et Applications I. Springer, Berlin, 1972.Google Scholar
[29] Lozanovskiĭ, G. Ya., On some Banach lattices. Sibirsk. Mat. Z. 10(1969), 584–599; English Transl. in Siberian. Math. J. 10(1969), 419–431.Google Scholar
[30] Lozanovskiĭ, G. Ya., On some Banach lattices IV. Sibirsk. Mat. Z. 14(1973), 140–155; English Transl. in Siberian. Math. J. 14(1973), 97–108.Google Scholar
[31] Lozanovskiĭ, G. Ya., Transformations of ideal Banach spaces by means of concave functions. In: Qualitative and Approximate Methods for the Investigation of Operator Equations, Yaroslav. Gos. Univ., Yaroslavl’ 1978, pp. 122–147 (Russian).Google Scholar
[32] Maligranda, L., Calderón–Lozanovskiĭ spaces and interpolation of operators. Semesterbericht Funktionalanalysis, Tübingen 8(1985), 83–92.Google Scholar
[33] Maligranda, L., On commutativity of interpolation with intersection. Rend. Circ. Mat. Palermo 10(1985), 113–118.Google Scholar
[34] Maligranda, L., A property of interpolation spaces. Arch. Math. 48(1987), 82–84.Google Scholar
[35] Maligranda, L., Orlicz Spaces and Interpolation. Sem. Math. 5, Univ. of Campinas, Campinas SP, Brazil, 1989.Google Scholar
[36] Maligranda, L., Why Hölder's inequality should be called Rogers’ inequality. Math. Inequal. Appl. 1(1998), 69–83.Google Scholar
[37] Maligranda, L., Positive bilinear operators in Calderón–Lozanovskiĭ spaces. Arch. Math. 81(2003), 26–37.Google Scholar
[38] Maligranda, L. and Persson, L. E., Generalized duality of some Banach function spaces. Indag.Math. 51(1989), 323–338.Google Scholar
[39] Muckenhoupt, B.,Weighted norm inequalities for the Hardy maximal function. Trans. Amer. Math. Soc. 165(1972), 207-226.Google Scholar
[40] Musielak, J., Orlicz Spaces and Modular Spaces. Lecture Notes in Math. 1034, Springer-Verlag, Berlin, 1983.Google Scholar
[41] Nilsson, P., Reiteration theorems for real interpolation and approximation spaces. Ann.Mat. Pura Appl. 132(1982), 291–330.Google Scholar
[42] Nilsson, P., Interpolation of Banach lattices. Studia Math. 82(1985), 135–154.Google Scholar
[43] Ovchinnikov, V. I., Interpolation theorems that arise from Grothendieck's inequality. Funktsional. Anal. i Prilozhen. (4) 10(1976), 45–54; English transl. in Functional Anal. Appl. 10(1976), 287–294 (1977).Google Scholar
[44] Ovchinnikov, V. I., The Methods of Orbits in Interpolation Theory. Math. Reports 1, Part 2, Harwood Academic Publishers 1984, 349–516.Google Scholar
[45] Pisier, G., Some applications of the complex interpolation method to Banach lattices. J. Analyse Math. 35(1979), 264–281.Google Scholar
[46] Reisner, S., Some remarks on Lozanovskyi's intermediate normed lattices. Bull. Polish Acad. Sci. Math. 41(1993), 189–196 (1994).Google Scholar
[47] Rochberg, R., Function theoretic results for complex interpolation families of Banach spaces. Trans. Amer.Math. Soc. 284(1984), 745–758.Google Scholar
[48] Rubio de Francia, J. L., A new technigue in the theory of Ap-weights. In: Topics in Modern Harmonic Analysis, Roma, 1983, pp. 571–579.Google Scholar
[49] Rubio de Francia, J. L., Factorization theory and Ap-weights. Amer. J. Math. 106(1984), 533–547.Google Scholar
[50] Shestakov, V. A., Transformations of Banach ideal spaces and interpolation of linear operators. Bull. Acad. Polon. Sci. Sér/ Math. 29(1981), 569–577 (1982) (Russian).Google Scholar
[51] Stafney, J. D., Analytic interpolation of certain multiplier spaces. Pacific J. Math. 32(1970), 241–248.Google Scholar
[52] Szeptycki, P., Notes on Integral Transformations. Dissertationes Math. (RozprawyMat.) 231, 1984.Google Scholar
[53] Triebel, H., Interpolation Theory, Function Spaces, Differential Operators. VEB Deutscher Verlag der Wissenschaften, Berlin, 1978.Google Scholar
[54] Wallstén, R., Remarks on interpolation of subspaces. In: Function Spaces and Applications, Lecture Notes in Math. 1302, Springer, Berlin, 1988, pp. 410–419.Google Scholar