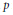Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-23T20:12:23.302Z Has data issue: false hasContentIssue false
  • English
  • Français

Reciprocity Law for Compatible Systems of Abelian mod $p$ Galois Representations

Published online by Cambridge University Press:  20 November 2018

Chandrashekhar Khare
Chandrashekhar Khare*
Affiliation:
Department of Mathematics, University of Utah, 155 S 1400 E, Salt Lake City, UT 84112, U.S.A., e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The main result of the paper is a reciprocity law which proves that compatible systems of semisimple, abelian mod 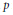$p$ representations (of arbitrary dimension) of absolute Galois groups of number fields, arise from Hecke characters. In the last section analogs for Galois groups of function fields of these results are explored, and a question is raised whose answer seems to require developments in transcendence theory in characteristic 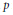$p$.

Keywords

11F80
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 57 , Issue 6 , 01 December 2005 , pp. 1215 - 1223
Copyright
Copyright © Canadian Mathematical Society 2005

References

[CS] Corrales-Rodrigánez, C., and Schoof, R., The support problem and its elliptic analogue. J. Number Theory 64(1997), 276–290.Google Scholar
[Go] Goss, D., Basic structures of function field arithmetic. Ergebnisse der Mathematik und ihrer Grenzgebiete 35, Springer-Verlag, Berlin, 1996.Google Scholar
[Gr] Gross, B., Algebraic Hecke characters for function fields. In: Seminar on Number Theory, Progr. Math. 22, Birkhauser, Boston, 1982, pp. 87–90 Google Scholar
[He] Henniart, G., Représentations ℓ-adiques abéliennes. In: Seminar on Number Theory, Progr. Math. 22 Birkhauser, Boston, 1982, pp. 107–126,.Google Scholar
[Kh] Khare, C., Compatible systems of mod p Galois representations and Hecke characters.Math. Res. Lett. 10(2003), 71–83.Google Scholar
[Se] Serre, J-P., Abelian ℓ–adic representations and elliptic curves. Second edition. Addison-Wesley, Redwood City, CA, 1989.Google Scholar