Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-26T00:45:09.578Z Has data issue: false hasContentIssue false
  • English
  • Français

Quotients jacobiens : une approche algébrique

Published online by Cambridge University Press:  20 November 2018

Carine Reydy
Carine Reydy*
Affiliation:
Laboratoire A2X, Institut de Mathématiques, 351, cours de la Libération, 33405 Talence, France email: [email protected]
Rights & Permissions [Opens in a new window]

Résumé

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Le diagramme d’Eisenbud et Neumann d’un germe est un arbre qui représente ce germe et permet d’en calculer les invariants. On donne une démonstration algébrique d’un résultat caractérisant l’ensemble des quotients jacobiens d’un germe d’application 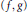$(f,\,g)$ à partir du diagramme d’Eisenbud et Neumann de 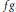$fg$.

Abstract

Abstract

The Eisenbud and Neumann diagram of a plane curve germ is a tree that represents this germ and allows computation of its invariants. We algebraically show a result that gives a caracterization of the set of jacobian quotients of an application germ 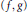$(f,\,g)$ for the datum of the Eisenbud et Neumann diagram of 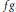$fg$.

Keywords

14B0532S0532S50Singularitéjacobienquotient jacobienpolygone de Newton
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 59 , Issue 5 , 01 October 2007 , pp. 1069 - 1097
Copyright
Copyright © Canadian Mathematical Society 2007

References

Références

[A] Abhyankar, S S., Lectures on Expansion Techniques in Algebraic Geometry. Notes by Balwant Singh. Tata Institute of Fundamental Research Lectures on Mathematics and Physics 57, Tata Institute of Fundamental Research, Bombay, 1977.Google Scholar
[BK] Brieskorn, E. et Knörrer, H., Plane Algebraic Curves. Birkhäuser Verlag, Basel, 1986.Google Scholar
[CA] Casas-Alvero, E., Singularities of plane curves. London Mathematical Society Lecture Note Series 276. Cambridge University Press, Cambridge, 2000.Google Scholar
[EN] Eisenbud, D. et Neumann, W. D.. Three-dimensional link theory and invariants of plane curve singularities. Annals of Mathematics Studies 110, Princeton University Press, Princeton, NJ, 1985.Google Scholar
[GB] García Barroso, Evelia R., Courbes polaires et courbure des fibres de Milnor des courbes planes. Thèse de doctorat. Université Paris-7, 2000.Google Scholar
[H] Heitmann, R. C., On the Jacobian conjecture. J. Pure Appl. Algebra 64(1990), 35–72.Google Scholar
[Hi] Hironaka, H., Introduction to the theory of infinitely near singular points. Memorias de Matematica del instituto Jorge Juan, 28, Consejo Superior de Investigaciones Cientficas, Madrid, 1974.Google Scholar
[KP] Kuo, T-C et Parusiński, A., On Puiseux roots of Jacobians. Proc. Japan Acad. Ser. A Math. Sci. 78(2002), no. 5, 55–59.Google Scholar
[L] Lê, D. T., Calcul du nombre de cycles évanouissants d’une hypersurface complexe. Ann. Inst. Fourier (Grenoble) 23(1973) no. 4, 261–270.Google Scholar
[LMW] Lê, D. T., Michel, F. et Weber, C., Sur le comportement des polaires associées aux germes de courbes planes. Compositio Math. 72(1989), no. 1, 87–113.Google Scholar
[M] Maugendre, H., Topologie des germes jacobiens. C. R. Acad. Sci. Paris Sér. I, 322(1996), 945–948.Google Scholar
[Me] Merle, M., Invariants polaires des courbes planes. Invent. Math. 41(1977), no. 2, 103–111.Google Scholar
[R] Reydy, C., Étude d’invariants des germes de courbes planes à l’aide des diagrammes de Newton. Thèse de doctorat, Université Bordeaux I, 2002.Google Scholar
[T] Teissier, B., Variétés polaires. I. Invariants polaires des singularités d’hypersurfaces. Invent.Math. 40(1977), no. 3, 267–292.Google Scholar