Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Iancu, Mihai
2015.
A Density Result for Parametric Representations in Several Complex Variables.
Computational Methods and Function Theory,
Vol. 15,
Issue. 2,
p.
247.
Iancu, Mihai
2016.
On Reachable Families of the Loewner Differential Equation in Several Complex Variables.
Complex Analysis and Operator Theory,
Vol. 10,
Issue. 2,
p.
353.
Bracci, Filippo
Graham, Ian
Hamada, Hidetaka
and
Kohr, Gabriela
2016.
Variation of Loewner Chains, Extreme and Support Points in the Class $$S^0$$ S 0 in Higher Dimensions.
Constructive Approximation,
Vol. 43,
Issue. 2,
p.
231.
Graham, Ian
Hamada, Hidetaka
Kohr, Gabriela
and
Kohr, Mirela
2016.
Support Points and Extreme Points for Mappings with $$A$$ A -Parametric Representation in $$\mathbb {C}^{n}$$ C n.
The Journal of Geometric Analysis,
Vol. 26,
Issue. 2,
p.
1560.
Hamada, Hidetaka
Iancu, Mihai
and
Kohr, Gabriela
2016.
Extremal Problems for Mappings with Generalized Parametric Representation in $${{\mathbb {C}}}^{n}$$ C n.
Complex Analysis and Operator Theory,
Vol. 10,
Issue. 5,
p.
1045.
Graham, Ian
Hamada, Hidetaka
Kohr, Gabriela
and
Kohr, Mirela
2017.
Bounded support points for mappings with g-parametric representation in C2.
Journal of Mathematical Analysis and Applications,
Vol. 454,
Issue. 2,
p.
1085.
Graham, Ian
Hamada, Hidetaka
Kohr, Gabriela
and
Kohr, Mirela
2017.
Geometric Function Theory in Higher Dimension.
Vol. 26,
Issue. ,
p.
165.
Roth, Oliver
2017.
Geometric Function Theory in Higher Dimension.
Vol. 26,
Issue. ,
p.
87.
Hamada, Hidetaka
Iancu, Mihai
and
Kohr, Gabriela
2017.
Geometric Function Theory in Higher Dimension.
Vol. 26,
Issue. ,
p.
117.
Hamada, H.
Iancu, M.
Kohr, G.
and
Schleißinger, S.
2018.
Approximation properties of univalent mappings on the unit ball in Cn
.
Journal of Approximation Theory,
Vol. 226,
Issue. ,
p.
14.
Bracci, Filippo
and
Roth, Oliver
2018.
Complex Analysis and Dynamical Systems.
p.
67.
Graham, Ian
Hamada, Hidetaka
and
Kohr, Gabriela
2018.
Complex Analysis and Dynamical Systems.
p.
141.
Schleißinger, Sebastian
2018.
On the parametric representation of univalent functions on the polydisc.
Rocky Mountain Journal of Mathematics,
Vol. 48,
Issue. 3,
Hamada, H.
Iancu, M.
and
Kohr, G.
2019.
Approximation of univalent mappings by automorphisms and quasiconformal diffeomorphismsin Cn.
Journal of Approximation Theory,
Vol. 240,
Issue. ,
p.
129.
Graham, Ian
Hamada, Hidetaka
and
Kohr, Gabriela
2020.
Loewner chains and nonlinear resolvents of the Carathéodory family on the unit ball in Cn.
Journal of Mathematical Analysis and Applications,
Vol. 491,
Issue. 1,
p.
124289.
Hamada, Hidetaka
and
Kohr, Gabriela
2020.
Support points for families of univalent mappings on bounded symmetric domains.
Science China Mathematics,
Vol. 63,
Issue. 12,
p.
2379.
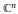 ${{\mathbb{C}}^{n}}$.
${{\mathbb{C}}^{n}}$.