Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-26T00:27:24.610Z Has data issue: false hasContentIssue false
  • English
  • Français

Polynomial Rings with the Outer Product Property

Published online by Cambridge University Press:  20 November 2018

A. V. Geramita
A. V. Geramita*
Affiliation:
Queen's University, Kingston, Ontario
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In [3] Lissner defined a class of rings called outer product rings, (OP-rings). These are commutative rings R with identity for which every exterior vector 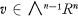is decomposable, i.e., 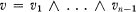with .

If we look only at those vectors whose co-ordinates with respect to any basis of 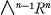generate the unit ideal in R and consider those rings R for which all vectors of this type are decomposable, we obtain the class of rings which have been referred to as Her mite-rings (H-rings, see also Lissner [3]). This class of H-rings evidently contains the class of OP-rings.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 24 , Issue 5 , October 1972 , pp. 866 - 872
Copyright
Copyright © Canadian Mathematical Society 1972

References

1. Auslander, M. and Buchsbaum, D., Homological dimension in local rings, Trans. Amer. Math. Soc. 85 (1957), 390–405.Google Scholar
2. Cohen, I. S., On the structure and ideal theory of complete local rings, Trans. Amer. Math. Soc. 59 (1946), 54–106.Google Scholar
3. Lissner, D., Outer product rings, Trans. Amer. Math. Soc. 116 (1965), 526–535.Google Scholar
4. Lissner, D., OPrings and Seshadri's theorem, J. Algebra 3 (1967), 362–366.Google Scholar
5. Lissner, D. and Geramita, A., Towber rings, J. Algebra 15 (1970), 13–40.Google Scholar
6. Northcott, D. G., An introduction to homological algebra (Cambridge University Press, Cambridge, London/New York, 1962).Google Scholar
7. Serre, J. P., Faisceaux algebriques coherents, Ann. of Math. 61 (1955), 197–278.Google Scholar
8. Serre, J. P., Modules projectif et espaces fibrés à fibre vectorielle (Séminaire Dubreil, 1957-58, exposé 23).Google Scholar
9. Serre, J. P., Sur les modules projectifs (Séminaire Dubreil-Pisot, Paris University, 1960-61, exposé 2).Google Scholar
10. Seshadri, C. S., Triviality of vector bundles over the affine space K2, Proc. Nat. Acad. Sci. U.S.A. U (1958), 456-458.Google Scholar
11. Swan, R. G., Algebraic K-theory (Lecture notes in Mathematics, No. 76, Springer-Verlag Berlin, 1968).Google Scholar
12. Towber, J., Complete reducibility in exterior algebras over free modules, J. Algebra 10 (1968), 299–309.Google Scholar
13. Towber, J., Local rings with the outer product property, Illinois J. Math. 14 (1970), 194–197.Google Scholar