Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-24T03:15:49.344Z Has data issue: false hasContentIssue false
  • English
  • Français

Partial Differential Hamiltonian Systems

Published online by Cambridge University Press:  20 November 2018

Luca Vitagliano
Luca Vitagliano*
Affiliation:
DipMat, University of Salerno, and Istituto Nazionale di Fisica Nucleare, GC Salerno, via Ponte don Melillo, 84084 Fisciano (SA)Italy, e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We define partial differential (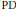$\text{PD}$ in the following), i.e., field theoretic analogues of Hamiltonian systems on abstract symplectic manifolds and study their main properties, namely, 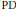$\text{PD}$ Hamilton equations, 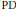$\text{PD}$ Noether theorem, 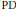$\text{PD}$ Poisson bracket, etc. Unlike the standard multisymplectic approach to Hamiltonian field theory, in our formalism, the geometric structure (kinematics) and the dynamical information on the “phase space” appear as just different components of one single geometric object.

Keywords

70S0570S1053C80field theoryfiber bundlesmultisymplectic geometryHamiltonian systems
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 65 , Issue 5 , 01 October 2013 , pp. 1164 - 1200
Copyright
Copyright © Canadian Mathematical Society 2013

References

[1] Aldaya, V., and de Azcárraga, J., Higher Order Hamiltonian Formalism in Field Theory. J. Phys. A: Math. Gen. 13(1982), 2545. http://dx.doi.org/10.1088/0305-4470/13/8/004 Google Scholar
[2] Alonso-Blanco, R. J. and Vinogradov, A. M., Green Formula and Legendre Transformation. Acta Appl. Math. 83(2004), 149. http://dx.doi.org/10.1023/B:ACAP.0000035594.33327.71 Google Scholar
[3] Awane, A., k-Symplectic Structures. J. Math. Phys. 32(1992), 4046. http://dx.doi.org/10.1063/1.529855 Google Scholar
[4] Bocharov, A. V., Chetverikov, V. N., Duzhin, S. V., Khor’kova, N. G., Krasil’shchik, I. S., Samokhin, A. V., Torkhov, Yu. N., Verbovetsky, A. M., and Vinogradov, A. M., Symmetries and Conservation Laws for Differential Equations of Mathematical Physics. Transl. Math. Mon. 182, Amer. Math. Soc., Providence, 1999.Google Scholar
[5] Bridges, T. J., Multi-symplectic Structures and Wave Propagation. Math. Proc. Camb. Philos. Soc. 121(1997), 147. http://dx.doi.org/10.1017/S0305004196001429 Google Scholar
[6] Bridges, T. J. and Reich, S., Multi-symplectic Integrators: Numerical Schemes for Hamiltonian PDEs that Preserve Symplecticity. Phys. Lett. A284(2001), 184. http://dx.doi.org/10.1016/S0375-9601(01)00294-8 Google Scholar
[7] Cantrijn, F. and Ibort, A.. de Lóen, M., On the Geometry of Multisymplectic Manifolds. J. Austral. Math. Soc. Ser. A 66(1999), 303. http://dx.doi.org/10.1017/S1446788700036636 Google Scholar
[8] Cotter, C. J., Holm, D. D., and Hydon, P. E., Multisymplectic Formulation of Fluid Dynamics Using the Inverse Map. Proc. Roy. Soc. A463(2007), 2671. chttp://dx.doi.org/10.1098/rspa.2007.1892 Google Scholar
[9] Crnković, C. and Witten, E., Covariant Description of Canonical Formalism in Geometrical Theories. In: Three Hundred Years of Gravitation (eds. S.W. Hawking andW. Israel), Cambridge University Press, Cambridge, 1987, 676.Google Scholar
[10] Dedecker, P., On the Generalization of Symplectic Geometry to Multiple Integrals in the Calculus of Variations. Lecture Notes in Math. 570, Springer, Berlin, 1977, 395.Google Scholar
[11] de Lecn, M., Marín-Solano, J., and Marrero, J. C., The Constraint Algorithm in the Jet Formalism. Diff. Geom. Appl. 6(1996), 275. http://dx.doi.org/10.1016/0926-2245(96)82423-5 Google Scholar
[12] de León, M., A Geometrical Approach to Classical Field Theories: a Constraint Algorithm for Singular Theories. Math. Appl. 350, Kluwer, Dordrecht, 1996, 291.Google Scholar
[13] de León, M., Marín-Solano, J., Marrero, J. C., Mu˜ñoz-Lecanda, M. C., and Román-Roy, N., Singular Lagrangian on Jet Bundles. Fort. Phys. 50(2002), 103. arxiv:math-ph/0105012Google Scholar
[14] de León, M., Martin de Diego, D., and Santamaria-Merino, A., Symmetries in Classical Field Theory. Int. J. Geom. Methods Mod. Phys. 1(2004), 651. http://dx.doi.org/10.1142/S0219887804000290 Google Scholar
[15] de León, M., Marín-Solano, J., Marrero, J. C., Mu˜ñoz-Lecanda, M. C., and Román-Roy, N., Pre-Multisymplectic Constraint Algorithm for Field Theories. Int. J. Geom. Methods Mod. Phys. 2(2005), 839. http://dx.doi.org/10.1142/S0219887805000880 Google Scholar
[16] Dubrovin, B. A. and Novikov, S. P., Hamiltonian Formalism of One-Dimensional Systems of Hydrodynamic Type and the Bogolyubov–Whitham Averaging Method. Dokl. Akad. Nauk SSSR 270(1983), 781–785; Soviet Math. Dokl. 27(1983), 665.Google Scholar
[17] Dubrovin, B. A., On Poisson Brackets of Hydrodynamic Type. Dokl. Akad. Nauk SSSR 279(1984), 294–297; Soviet Math. Dokl. 30(1984), 651.Google Scholar
[18] Echeverría-Enríquez, A., Mu˜ñoz-Lecanda, M. C., and N. Román-Roy, , Geometry of Multisymplectic Hamiltonian First-Order Field Theories. J. Math. Phys. 41(2000), 7402. http://dx.doi.org/10.1063/1.1308075 Google Scholar
[19] Echeverría-Enríquez, A., Geometry of Lagrangian First-Order Classical Field Theories. Forts. Phys. 44(1996), 235. http://dx.doi.org/10.1002/prop.2190440304 Google Scholar
[20] Forger, M. and Gomes, L., Multisymplectic and Polysymplectic Structures on Fiber Bundles. arxiv:0708.1596Google Scholar
[21] Forger, M., Paufler, C., and Römer, H., The Poisson Bracket for Poisson Forms in Multisymplectic Field Theory. Rev. Math. Phys. 15(2003), 705. http://dx.doi.org/10.1142/S0129055X03001734 Google Scholar
[22] Forger, M., A General Construction of Poisson Brackets on Exact Multisymplectic Manifolds. Rep. Math. Phys. 51(2003), 187. http://dx.doi.org/10.1016/S0034-4877(03)80012-5 Google Scholar
[23] Forger, M., Hamiltonian Multivector Fields and Poisson Forms in Multisymplectic Field Theory. J. Math. Phys. 46(2005), 112903. http://dx.doi.org/10.1063/1.2116320 Google Scholar
[24] Forger, M. and Römer, H., A Poisson Bracket on Multisymplectic Phase Space. Rep. Math. Phys. 48(2001), 211. http://dx.doi.org/10.1016/S0034-4877(01)80081-1 Google Scholar
[25] Forger, M. and Romero, S., Covariant Poisson Brackets in Geometric Field Theory. Commun. Math. Phys. 256(2005), 375. http://dx.doi.org/10.1007/s00220-005-1287-8 Google Scholar
[26] Goldshmidt, H. and Sternberg, S., The Hamilton–Cartan Formalism in the Calculus of Variations. Ann. Inst. Fourier 23(1973), 203. http://dx.doi.org/10.5802/aif.451 Google Scholar
[27] Gotay, M. J., A Multisymplectic Approach to the KdV Equation. In: Differential Geometric Methods in Mathematical Physics (eds. K. Bleuler and M.Werner), Kluwer, Amsterdam, 1988, 295.Google Scholar
[28] Gotay, M. J., Isenberg, J., and Marsden, J. E., Momentum Maps and Classical Relativistic Fields. I: Covariant Field Theory. arxiv:physics/9801019Google Scholar
[29] Gotay, M. J., Nester, J. M., and Hinds, G., Presymplectic Manifolds and the Dirac–Bergmann Theory of Constraints. J. Math. Phys. 19(1978), 2388. http://dx.doi.org/10.1063/1.523597 Google Scholar
[30] Grabowska, K., A Tulczyjew Triple for Classical Fields. J. Phys. A: Math. Theor. 45(2012), 145207. http://dx.doi.org/10.1088/1751-8113/45/14/145207 Google Scholar
[31] Grabowska, K., Grabowski, J., and Urbański, P., AV-Differential Geometry: Poisson and Jacobi Structures. J. Geom. Phys. 52(2004), 398. http://dx.doi.org/10.1016/j.geomphys.2004.04.004 Google Scholar
[32] Grabowska, K., AV-Differential Geometry: Euler–Lagrange Equations. J. Geom. Phys. 57(2007), 1984. http://dx.doi.org/10.1016/j.geomphys.2007.04.003 Google Scholar
[33] Gracia, X., Martin, R., and Román-Roy, N., Constraint Algorithm for k-Presymplectic Hamiltonian Systems. Application to Singular Field Theories. Int. J. Geom. Methods Mod. Phys. 6(2009), 851. http://dx.doi.org/10.1142/S0219887809003795 Google Scholar
[34] Hélein, F. and Kouneiher, J., Covariant Hamiltonian Formalism for the Calculus of Variations with Several Variables: Lepage–Dedecker versus De Donder–Weyl. Adv. Theor. Math. Phys. 8(2004), 565.Google Scholar
[35] Henneaux, M. and Teitelboim, C., Quantization of Gauge Systems. Princeton University Press, Princeton, 1992.Google Scholar
[36] Kanatchikov, I. V., On Field Theoretic Generalization of a Poisson Algebra. Rep. Math. Phys. 40(1997), 225. http://dx.doi.org/10.1016/S0034-4877(97)85919-8 Google Scholar
[37] Kijowski, J., A Finite-Dimensional Canonical Formalism in the Classical Field Theory. Commun. Math. Phys. 30(1973), 99. http://dx.doi.org/10.1007/BF01645975 Google Scholar
[38] Kijowski, J. and Szczyrba, W., Multisymplectic Manifolds and the Geometrical Construction of the Poisson Bracket in Field Theory. In: Géométrie Symplectique et Physique Mathématique (ed. J.-M. Souriau), Colloq. Internat. C. N. R. S. 237(1975), 347.Google Scholar
[39] Kolář, I., A Geometric Version of the Higher Order Hamilton Formalism in Fibered Manifolds. J. Geom. Phys. 1(1984), 127. http://dx.doi.org/10.1016/0393-0440(84)90007-X Google Scholar
[40] Krupkova, O., Hamiltonian Field Theory. J. Geom. Phys. 43(2002), 93. http://dx.doi.org/10.1016/S0393-0440(01)00087-0 Google Scholar
[41] Lee, J. and Wald, R., Local Symmetries and Constraints. J. Math. Phys. 31(1990), 725. http://dx.doi.org/10.1063/1.528801Google Scholar
[42] Marsden, J., Pekarsky, S., Shkoller, S., and West, M., Variational Methods, Multisymplectic Geometry and Continuum Mechanics. J. Geom. Phys. 38(2001), 253. http://dx.doi.org/10.1016/S0393-0440(00)00066-8 Google Scholar
[43] Martin, G., A Darboux Theorem for Multisymplectic Manifolds. Lett. Math. Phys. 16(1988), 133.http://dx.doi.org/10.1007/BF00402020 Google Scholar
[44] Michor, P.W., Topics in Differential Geometry. Graduate Stud. in Math. 93, Amer. Math. Soc., Providence, 2008.Google Scholar
[45] Mokhov, O. I., Symplectic and Poisson Geometry on Loop Spaces of Manifolds and Nonlinear Equations. Uspekhi Mat. Nauk 53(1998), 85–192; (English) Russian Math. Surveys 53(1998), 515.http://dx.doi.org/10.4213/rm19 Google Scholar
[46] Moreno, G., Vinogradov, A. M., and Vitagliano, G., Integrals and Cohomology. In preparation.Google Scholar
[47] Paufler, C. and Römer, H., Geometry of Hamiltonian n-Vectors in Multisymplectic Field Theory. J. Geom. Phys. 44(2002), 52. http://dx.doi.org/10.1016/S0393-0440(02)00031-1 Google Scholar
[48] Paufler, C., de Donder–Weyl Equations and Multisymplectic Geometry. Rep. Math. Phys. 49(2002), 325. http://dx.doi.org/10.1016/S0034-4877(02)80030-1 Google Scholar
[49] Román-Roy, N., Multisymplectic Lagrangian and Hamiltonian Formalism of First-Order Classical Field Theories. SIGMA 5(2009), 100.arxiv:math-ph/0506022Google Scholar
[50] Saunders, D. J., Jet Fields, Connections and Second-Order Differential Equations. J. Phys. A: Math. Gen. 20(1987), 3261.http://dx.doi.org/10.1088/0305-4470/20/11/029 Google Scholar
[51] Saunders, D. J., The Geometry of Jet Bundles. Cambridge University Press, Cambridge, 1989.Google Scholar
[52] Saunders, D. J., A Note on Legendre Transformations. Diff. Geom. Appl. 1(1991), 109. http://dx.doi.org/10.1016/0926-2245(91)90025-5Google Scholar
[53] Saunders, D. J. and Crampin, M., On the Legendre Map in Higher-Order Field Theories. J. Phys. A: Math. Gen. 23(1990), 3169. http://dx.doi.org/10.1088/0305-4470/23/14/016 Google Scholar
[54] Shadwick, W. F., The Hamiltonian Formulation of Regular r-th Order Lagrangian Field Theories. Lett. Math. Phys. 6(1982), 409.http://dx.doi.org/10.1007/BF00405859 Google Scholar
[55] Vinogradov, A. M., The –Spectral Sequence, Lagrangian Formalism and Conservation Laws I, II. J. Math. Anal. Appl. 100(1984), 1.http://dx.doi.org/10.1016/0022-247X(84)90071-4 Google Scholar
[56] Vitagliano, L., Secondary Calculus and the Covariant Phase Space. J. Geom. Phys. 59(2009), 426.http://dx.doi.org/10.1016/j.geomphys.2008.12.001 Google Scholar
[57] Vitagliano, L., The Lagrangian–Hamiltonian Formalism for Higher Order Field Theories. J. Geom. Phys. 60(2010), 857.http://dx.doi.org/10.1016/j.geomphys.2010.02.003 Google Scholar
[58] Zuckerman, G. J., Action Principles and Global Geometry. In: Mathematical Aspects of String Theory (ed. S. T. Yau),World Scientific, Singapore, 1987, 259.Google Scholar