Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-19T17:35:40.932Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Symmetries of Spherical Harmonics

Published online by Cambridge University Press:  20 November 2018

Burnett Meyer
Burnett Meyer*
Affiliation:
University of Arizona
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

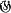Let be a finite group of transformations of three-dimensional Euclidean space, such that the distance between any two points is preserved by all transformations of the group. Such a group is a group of orthogonal linear transformations of three variables, or, geometrically speaking, a group of rotations and rotatory inversions. Thirty-two groups of this type are important in crystallography and are known as the crystallographic classes.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 6 , 1954 , pp. 135 - 157
Copyright
Copyright © Canadian Mathematical Society 1954

References

1. Bethe, H., Termaufspaltung in Kristallen, Ann. Phys. (5), 3, (1929) 133–208.Google Scholar
2. Burnside, W., Theory of groups of finite order, 2nd ed. (Cambridge, 1911).Google Scholar
3. Coxeter, H. S. M., Regular polytopes (New York, 1948).Google Scholar
3a. Coxeter, H. S. M., The product of the generators of a finite group generated by reflections, Duke Math. J., 18 (1951), 765–782.Google Scholar
4. Ehlert, W., Über das Schwingungs–und Rotation sspektrum einer Molekel vont Typus CH4. Z. Phys. 51 (1928) 6–33.Google Scholar
5. Hobson, E. W., The theory of spherical and ellipsoidal harmonics (Cambridge, 1931).Google Scholar
6. Hodgkinson, J., Harmonic functions with polyhedral symmetry, J. London Math. Soc, 10 (1935), 221–226.Google Scholar
7. Laporte, O., Polyhedral harmonics, Z. Naturforsch., 3a (1948), 447–456.Google Scholar
8. Macduffee, C. C., The theory of matrices (Berlin, 1933).Google Scholar
9. Molien, T., Über die Invarianten der linear en Substitutionsgruppen, S. B. Akad. VViss. Berlin, 2 (1897), 1152–1156.Google Scholar
10. Pólya, G. and Meyer, B., Sur les symétries des fonctions sphêriques de Laplace, C. R. Acad. Sci., Paris, 228 (1949), 28–30.Google Scholar
11. Pólya, G. and Meyer, B., Sur les fonctions sphêriques de Laplace de symétrie cristallo graphique donnée, C.R. Acad. Sci., Paris, 228 (1949), 1083–1084.Google Scholar
12. Pólya, G. and Szegö, G., Isoperimetric inequalities in mathematical physics (Princeton, 1951).Google Scholar
13. Poole, E. G. C., Spherical harmonies having polyhedral symmetry, Proc. London Math. Soc. (2), 33 (1932), 435–456.Google Scholar
14. Speiser, A., Die Theorie der Gruppen von endlicher Ordnung (New York, 1945).Google Scholar
15. Stiefel, E., Two applications of group characters to the solution of boundary-value problems, J. Res. Nat. Bur. Standards, 48 (1952), 424–427.Google Scholar
16. Turnbull, H. W., The theory of determinants, matrices, and invariants (London, 1928).Google Scholar
17. Weyl, H., Symmetry (Princeton, 1952).Google Scholar