Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-26T12:15:48.093Z Has data issue: false hasContentIssue false
  • English
  • Français

On Kaehler Immersions

Published online by Cambridge University Press:  20 November 2018

Koichi Ogiue
Koichi Ogiue*
Affiliation:
Tokyo Metropolitan University, Tokyo, Japan
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

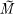Let be an (n + p)-dimensional Kaehler manifold of constant holomorphic sectional curvature . B. O'Neill [3] proved the following result.

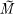PROPOSITION A. Let M be an n-dimensional complex submanifold immersed in . If 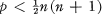and if the holomorphic sectional curvature of M with respect to the induced Kaehler metric is constant, then M is totally geodesic.

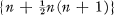He also gave the following example: There is a Kaehler imbedding of an w-dimensional complex projective space of constant holomorphic sectional curvature ½ into an -dimensional complex projective space of constant holomorphic sectional curvature 1. This shows that Proposition A is the best possible.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 24 , Issue 6 , December 1972 , pp. 1178 - 1182
Copyright
Copyright © Canadian Mathematical Society 1972

References

1. Ogiue, K., Differential geometry of algebraic manifolds, Differential Geometry, in honor of Yano, K., 355-372 (Kinokuniya, Tokyo, 1972).Google Scholar
2. Ogiue, K., Positively curved complex submanifolds immersed in a complex projective space (to appear in J. Differential Geometry).Google Scholar
3. O'Neill, B., Isotropic and Kaehler immersions, Can. J. Math. 17 (1965), 907–915.Google Scholar