Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Lambek, Joachim
and
Moser, Leo
1955.
On Integers n Relatively Prime To ƒ(n).
Canadian Journal of Mathematics,
Vol. 7,
Issue. ,
p.
155.
Erdős, P.
1958.
Asymptotic Formulas for Some Arithmetic Functions.
Canadian Mathematical Bulletin,
Vol. 1,
Issue. 3,
p.
149.
Fainleib, A. S.
1972.
On the relative primality of n andf (n).
Mathematical Notes of the Academy of Sciences of the USSR,
Vol. 11,
Issue. 3,
p.
163.
Erdös, P.
and
Lorentz, G. G.
1997.
Mathematics from Leningrad to Austin.
p.
252.
Erdös, P.
and
Lorentz, G. G.
1997.
Mathematics from Leningrad to Austin.
p.
252.
NOGUEIRA, ARNALDO
2010.
Lattice orbit distribution on ℝ2.
Ergodic Theory and Dynamical Systems,
Vol. 30,
Issue. 4,
p.
1201.
Bergelson, Vitaly
and
Richter, Florian Karl
2017.
Number Theory – Diophantine Problems, Uniform Distribution and Applications.
p.
109.
Ding, Yuchen
2022.
On the numbers 𝑛 relatively prime to Ω(𝑛)-𝜔(𝑛).
Proceedings of the American Mathematical Society,
Vol. 150,
Issue. 9,
p.
3811.
Banks, William
and
Shparlinski, Igor E.
2024.
On the Greatest Common Divisor of Integer Parts of Polynomials.
Michigan Mathematical Journal,
Vol. -1,
Issue. -1,


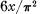 (or in other words that they have the density
(or in other words that they have the density 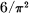 ), for irrational a. I prove this and rather more in the following
), for irrational a. I prove this and rather more in the following