Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-27T19:48:18.979Z Has data issue: false hasContentIssue false
  • English
  • Français

On Effective Witt Decomposition and the Cartan–Dieudonné Theorem

Published online by Cambridge University Press:  20 November 2018

Lenny Fukshansky
Lenny Fukshansky*
Affiliation:
Department of Mathematics, Mailstop 3368, Texas A&M University, College Station, TX 77843-3368 email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let $K$ be a number field, and let 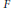$F$ be a symmetric bilinear form in 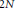$2N$ variables over $K$. Let 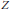$Z$ be a subspace of 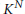${{K}^{N}}$. A classical theorem of Witt states that the bilinear space 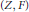$\left( Z,\,F \right)$ can be decomposed into an orthogonal sum of hyperbolic planes and singular and anisotropic components. We prove the existence of such a decomposition of small height, where all bounds on height are explicit in terms of heights of 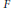$F$ and 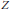$Z$. We also prove a special version of Siegel's lemma for a bilinear space, which provides a small-height orthogonal decomposition into one-dimensional subspaces. Finally, we prove an effective version of the Cartan-Dieudonné theorem. Namely, we show that every isometry $~\sigma$ of a regular bilinear space 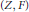$\left( Z,\,F \right)$ can be represented as a product of reflections of bounded heights with an explicit bound on heights in terms of heights o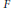f $F$, 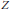$Z$, and $~\sigma$.

Keywords

11E1215A6311G50quadratic formsheights
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 59 , Issue 6 , 01 December 2007 , pp. 1284 - 1300
Copyright
Copyright © Canadian Mathematical Society 2007

References

[1] Bombieri, E. and Vaaler, J. D., On Siegel's lemma. Invent. Math. 73(1983), no. 1, 11–32.Google Scholar
[2] Cassels, J. W. S., Bounds for the least solutions of homogeneous quadratic equations. Proc. Cambridge Philos. Soc. 51(1955), 262–264.Google Scholar
[3] Fukshansky, L., Small zeros of quadratic forms with linear conditions. J. Number Theory 108(2004), no. 1, 29–43.Google Scholar
[4] Gordan, P., Uber den grossten gemeinsamen factor. Math. Ann. 7(1873), 443–448.Google Scholar
[5] Hodge, W. V. D. and Pedoe, D., Methods of Algebraic Geometry. Vol. 1, Cambridge at the University Press, New York, 1947.Google Scholar
[6] Masser, D. W., How to solve a quadratic equation in rationals. Bull. London Math. Soc. 30(1998), no. 1, 24–28.Google Scholar
[7] Masser, D. W., Search bounds for Diophantine equations. In: A Panorama of Number Theory or the View from Baker's Garden. Cambridge University Press, Cambridge, pp. 247–259, 2002.Google Scholar
[8] O’Meara, O. T., Introduction to Quadratic Forms. Second Printing, corrected. Grundlehren der Mathematischen Wissenschaften 117, Springer-Verlag, New York, 1971.Google Scholar
[9] Roy, D. and Thunder, J. L., An absolute Siegel's lemma. J. Reine Angew. Math. 476(1996), 1–26.Google Scholar
[10] Scharlau, W., Quadratic and Hermitian Forms. Grundlehren der Mathematischen Wissenschaften 270, Springer-Verlag, Berlin, 1985.Google Scholar
[11] Schlickewei, H. P. and Schmidt, W. M.. Quadratic geometry of numbers. Trans. Amer. Math. Soc. 301(1987), no. 2, 679–690.Google Scholar
[12] Struppeck, T. and Vaaler, J. D., Inequalities for heights of algebraic subspaces and the Thue-Siegel principle. Progr. Math. 85(1990), 493–528.Google Scholar
[13] Vaaler, J. D., Small zeros of quadratic forms over number fields. Trans. Amer. Math. Soc. 302(1987), no. 1, 281–296.Google Scholar
[14] Vaaler, J. D., Small zeros of quadratic forms over number fields. II. Trans. Amer. Math. Soc. 313(1989), no. 2, 671–686.Google Scholar