Article contents
A Note on Young's Raising Operator
Published online by Cambridge University Press: 20 November 2018
Extract
Consider the following formula due to Young [7] for the calculation of the homogeneous product sum, hλ, in terms of Schur functions;

where the operation Srs is defined as follows:
Y1: Srs, where r < s, “represents the operation of moving one letter from the s-th row up to the r-th row; and the resulting term is regarded as zero, when any row becomes less than a row below it, or when letters from the same row overlap; as, for instance, happens when λ1 = λ2 in the case of S13S23.“
The following example of the above is given by Robinson [4].
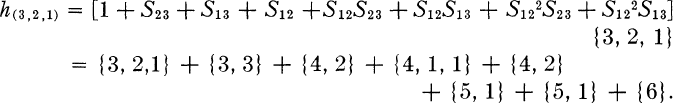
Calculation by other means shows that the above analysis of h(3,2,1) is correct; however, it will be noticed that the operator S123S23 does not appear in the above yet it is not specifically excluded by the rule Y1.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1981
References
- 5
- Cited by


