Published online by Cambridge University Press: 20 November 2018
In (3), Isbell proposed a stronger definition for the term “complete category” and obtained many nice theorems for the resulting notion of a completion. In particular, he showed (3, Theorem 3.20) that completions of small categories satisfy a strong normality condition.
In this paper we shall always use the term “complete” in the weaker sense of Freyd (1). (In (3), Isbell used the term “small-complete” for this weaker notion.) We shall prove that the completions, in the sense of Freyd, of small categories also enjoy the same normality condition, provided they admit at least one bicategory structure. (The complete categories in the sense of Isbell always admit bicategory structures; see the remark following Proposition 2.4.)
In what follows, we let  mean that
mean that 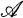 is a full subcategory of
is a full subcategory of  . Moreover, if
. Moreover, if  , then
, then 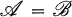 means that each object of
means that each object of  is equivalent to an object in
is equivalent to an object in 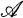 .
.