Hostname: page-component-745bb68f8f-v2bm5 Total loading time: 0 Render date: 2025-01-11T05:20:14.964Z Has data issue: false hasContentIssue false
  • English
  • Français

Non-tangential Maximal Function Characterizations of Hardy Spaces Associated with Degenerate Elliptic Operators

Published online by Cambridge University Press:  20 November 2018

Junqiang Zhang ,
Jun Cao ,
Renjin Jiang  and
Dachun Yang
Junqiang Zhang
Affiliation:
School of Mathematical Sciences, Beijing Normal University, Laboratory of Mathematics and Complex Systems, Ministry of Education, Beijing 100875, P. R. China. [email protected], [email protected], [email protected]
Jun Cao
Affiliation:
Department of Applied Mathematics, Zhejiang University of Technology, Hangzhou 310032, P. R. China. [email protected]
Renjin Jiang
Affiliation:
School of Mathematical Sciences, Beijing Normal University, Laboratory of Mathematics and Complex Systems, Ministry of Education, Beijing 100875, P. R. China. [email protected], [email protected], [email protected]
Dachun Yang
Affiliation:
School of Mathematical Sciences, Beijing Normal University, Laboratory of Mathematics and Complex Systems, Ministry of Education, Beijing 100875, P. R. China. [email protected], [email protected], [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 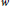$w$ be either in the Muckenhoupt class of 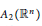${{A}_{2}}\left( {{\mathbb{R}}^{n}} \right)$ weights or in the class of 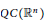$QC\left( {{\mathbb{R}}^{n}} \right)$ weights, and let 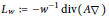${{L}_{w}}\,:=\,-{{w}^{-1}}\,\text{div}\left( A\nabla \right)$ be the degenerate elliptic operator on the Euclidean space ${{\mathbb{R}}^{n}}$, 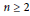$n\,\ge \,2$. In this article, the authors establish the non-tangential maximal function characterization of the Hardy space 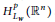$H_{{{L}_{w}}}^{p}\,\left( {{\mathbb{R}}^{n}} \right)$ associated with 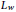${{L}_{w}}$ for 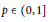$p\,\in \,(0,\,1]$, and when 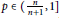$p\,\in \,(\frac{n}{n+1},\,1]$ and 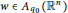$w\,\in \,{{A}_{{{q}_{0}}}}\left( {{\mathbb{R}}^{n}} \right)$ with 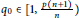${{q}_{0}}\,\in \,[1,\,\frac{p(n+1)}{n})$, the authors prove that the associated Riesz transform $\nabla L_{w}^{-1/2}$ is bounded from 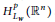$H_{{{L}_{w}}}^{p}\,\left( {{\mathbb{R}}^{n}} \right)$ to the weighted classical Hardy space 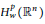$H_{w}^{p}\left( {{\mathbb{R}}^{n}} \right)$.

Keywords

42B2542B3042B3535J70degenerate elliptic operatorHardy spacesquare functionmaximal functionmoleculeRiesz transform
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 67 , Issue 5 , 01 October 2015 , pp. 1161 - 1200
Copyright
Copyright © Canadian Mathematical Society 2015

References

[1] Auscher, P.and Martell, J. M., Weighted norm inequalities, off-diagonal estimates and elliptic operators. II. Off-diagonal estimates on spaces of homogeneous type. J. Evol. Equ. 7(2007), no. 2, 265–316.http://dx.doi.org/10.1007/s00028-007-0288-9 Google Scholar
[2] Auscher, P., Mcintosh, A., and Russ, E., Hardy spaces of differential forms on Riemannian manifolds. J. Geom. Anal. 18(2008), no. 1,192–248.http://dx.doi.Org/10.1007/s12220-007-9003-x Google Scholar
[3] Bui, T. A., Cao, J., Ky, L. D., Yang, D., and Yang, S., Weighted Hardy spaces associated with operators satisfying reinforced off-diagonal estimates. Taiwanese J. Math. 17(2013), no. 4, 1127–1166.Google Scholar
[4] Bui, T. A., Cao, J., Ky, L. D., Yang, D., and Yang, S., Musielak-Orlicz-Hardy spaces associated with operators satisfying reinforced off-diagonal estimates. Anal. Geom. Metr. Spaces 1(2013), 69–129.Google Scholar
[5] Bui, T. A. and Li, J., Orlicz-Hardy spaces associated to operators satisfying bounded H∞ functional calculus and Davies-Gaffney estimates. J. Math. Anal. Appl. 373(2011), no. 2, 485–501.http://dx.doi.Org/10.1016/j.jmaa.2O10.07.050 Google Scholar
[6] Cao, J., Chang, D.-C., Yang, D., and Yang, S., Weighted local Orlicz-Hardy spaces on domains and their applications in inhomogeneous Dirichlet and Neumann problems. Trans. Amer. Math. Soc. 365(2013), no. 9, 4729–4809.http://dx.doi.org/10.1090/S0002-9947-2013-05832-1 Google Scholar
[7] Chanillo, S. and Wheeden, R. L., Some weighted norm inequalities for the area integral. Indiana Univ. Math. J. 36(1987), no. 2, 277–294.http://dx.doi.Org/10.1512/iumj.1987.36.36016 Google Scholar
[8] Chiarenza, F. and Franciosi, M., Quasiconformal mappings and degenerate elliptic and parabolic equations. Matematiche (Catania) 42(1987), no. 1–2,163–170.Google Scholar
[9] Chiarenza, F. and Frasca, M., Boundedness for the solutions of a degenerate parabolic equation. Applicable Anal. 17(1984), no. 4, 243–261.http://dx.doi.org/10.1080/00036818408839500 Google Scholar
[10] Chiarenza, F. and Serapioni, R., A remark on a Harnack inequality for degenerate parabolic equations. Rend. Sem. Mat. Univ. Padova 73(1985), 179–190.Google Scholar
[11] Coifman, R. R., Meyer, Y. and Stein, E. M., Some new function spaces and their applications to harmonic analysis. J. Funct. Anal. 62(1985), no. 2, 304–335.http://dx.doi.Org/10.1016/0022-1236(85)90007-2 Google Scholar
[12] Cruz-Uribe, D. and Rios, C., Gaussian bounds for degenerate parabolic equations. J. Funct. Anal. 255(2008), no. 2, 283–312. http://dx.doi.Org/10.1016/j.jfa.2008.01.017 Google Scholar
[13] Cruz-Uribe, D. and Rios, C., The solution of the Kato problem for degenerate elliptic operators with Gaussian bounds. Trans. Amer. Math. Soc. 364(2012), no. 7, 3449–3478.http://dx.doi.org/10.1090/S0002-9947-2012-05380-3 Google Scholar
[14] Cruz-Uribe, D. and Rios, C., The Kato problem for operators with weighted degenerate ellipticity. Trans. Amer. Math. Soc, to appear.http://dx.doi.org/10.1090/S0002-9947-2012-05380-3 Google Scholar
[15] Davies, E. B., Uniformly elliptic operators with measurable coefficients. J. Funct. Anal. 132(1995), no. 1, 141–169. http://dx.doi.org/10.1006/jfan.1995.1103 Google Scholar
[16] Duoandikoetxea, J., Fourier analysis. Graduate Studies in Mathematics, 29, American Mathematical Society, Providence, RI, 2001.Google Scholar
[17] Duong, X. T., Hofmann, S., Mitrea, D., Mitrea, M., and Yan, L., Hardy spaces and regularity for the inhomogeneous Dirichlet and Neumann problems. Rev. Mat. Iberoam. 29(2013), no. 1,183–236.http://dx.doi.Org/10.4171/RMI/718 Google Scholar
[18] Duong, X. T. and Li, J., Hardy spaces associated to operators satisfying Davies-Gaffney estimates and bounded holomorphic functional calculus. J. Funct. Anal. 264(2013), no. 6,1409–1437.http://dx.doi.0rg/IO.IOI6/j.jfa.2Oi3.OI.OO6 Google Scholar
[19] Duong, X. T. and Yan, L., New function spaces of BMO type, the fohn-Nirenberg inequality, interpolation, and applications. Comm. Pure Appl. Math. 58(2005), no. 10,1375–1420.http://dx.doi.Org/10.1OO2/cpa.2OO8O Google Scholar
[20] Duong, X. T. and Yan, L., Duality of Hardy and BMO spaces associated with operators with heat kernel bounds. J. Amer. Math. Soc. 18(2005), no. 4, 943–973.http://dx.doi.Org/10.1090/S0894-0347-05-00496-0 Google Scholar
[21] Fabes, E. B., Kenig, C. E., and Serapioni, R. P., The local regularity of solutions of degenerate elliptic equations. Comm. Partial Differential Equations 7(1982), 77–116.http://dx.doi.org/10.1080/03605308208820218 Google Scholar
[22] Fefferman, C. and Stein, E. M., Hp spaces of several variables. Acta Math. 129(1972), no. 3–4,137–193. http://dx.doi.org/10.1007/BF02392215 Google Scholar
[23] García-Cuerva, J., Weighted HP spaces. Dissertationes Math. (RozprawyMat.) 162(1979).Google Scholar
[24] Gehring, F. W., The Lp-integrability of the partial derivatives of a quasiconformal mapping. Acta Math. 130(1973), 265–277.http://dx.doi.org/10.1007/BF02392268 Google Scholar
[25] Hofmann, S., Lu, G., Mitrea, D., Mitrea, M., and Yan, L., Hardy spaces associated to non-negative self-adjoint operators satisfying Davies-Gaffney estimates. Mem. Amer. Math. Soc. 214(2011), no. 1007.Google Scholar
[26] Hofmann, S. and Martell, J. M., Lp bounds for Riesz transforms and square roots associated to second order elliptic operators. Publ. Mat. 47(2003), no. 2, 497–515. http://dx.doi.Org/10.5565/PUBLMAT_47203_12 Google Scholar
[27] Hofmann, S. and Mayboroda, S., Hardy and BMO spaces associated to divergence form elliptic operators. Math. Ann. 344(2009), no. 1, 37–116.http://dx.doi.org/10.1007/s00208-008-0295-3 Google Scholar
[28] Hofmann, S., Mayborod,a, S. and Mcintosh, A., Second order elliptic operators with complex bounded measurable coefficients in Lp, Sobolev and Hardy spaces. Ann. Sci. Éc. Norm. Super. (4)44(2011), no. 5, 723–800.Google Scholar
[29] Ishige, K., On the behavior of the solutions of degenerate parabolic equations. Nagoya Math. J. 155(1999), 1–26.Google Scholar
[30] Jiang, R., Cheeger-harmonic functions in metric measure spaces revisited. J. Funct. Anal. 266(2014), no. 3, 1373–1394. http://dx.doi.Org/10.1016/j.jfa.2013.11.022 Google Scholar
[31] Jiang, R. and Yang, D., New Orlicz-Hardy spaces associated with divergence form elliptic operators. J. Funct. Anal. 258(2010), no. 4, 1167–1224.http://dx.doi.Org/10.1016/j.jfa.2009.10.018 Google Scholar
[32] Jiang, R. and Yang, D., Orlicz-Hardy spaces associated with operators satisfying Davies-Gaffney estimates. Commun. Contemp. Math. 13(2011), no. 2, 331–373.http://dx.doi.Org/10.1142/S0219199711004221 Google Scholar
[33] Mcintosh, A., Operators which have an H∞ functional calculus. In: Miniconference on operator theory and partial differential equations (North Ryde, 1986), Proc. Centre Math. Anal. Austral. Nat. Univ., 14, Austral. Nat. Univ., Canberra, 1986, pp. 210–231.Google Scholar
[34] Ouhabaz, E. M., Analysis of heat equations on domains. London Mathematical Society Monographs Series, 31, Princeton University Press, Princeton, NJ, 2005.Google Scholar
[35] Russ, E., The atomic decomposition for tent spaces on spaces of homogeneous type. In: CMA/AMSI Research Symposium “Asymptotic Geometric Analysis, Harmonic Analysis, and Related Topics”, Proc. Centre Math. Appl. Austral. Nat. Univ., 42, Austral. Nat. Univ., Canberra, 2007, pp. 125–135.Google Scholar
[36] Stein, E. M., Singular integrals and differentiability properties of functions. Princeton Mathematical Series, 30, Princeton University Press, Princeton, NJ, 1970.Google Scholar
[37] Stein, E. M. and Weiss, G., On the theory of harmonic functions of several variables. I. The theory of Hp-spaces. Acta Math. 103(1960), 25–62.http://dx.doi.org/10.1007/BF02546524 Google Scholar
[38] Yan, L., Classes of Hardy spaces associated with operators, duality theorem and applications. Trans. Amer. Math. Soc. 360(2008), no. 8, 4383–4408.http://dx.doi.org/10.1090/S0002-9947-08-04476-0 Google Scholar