Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-24T16:35:16.531Z Has data issue: false hasContentIssue false
  • English
  • Français

Non-reductive Homogeneous Pseudo-Riemannian Manifolds of Dimension Four

Published online by Cambridge University Press:  20 November 2018

M. E. Fels  and
A. G. Renner
M. E. Fels
Affiliation:
Department of Mathematics and Statistics, Utah State University, Logan, UT 84322, U.S.A. e-mail: [email protected]@cc.usu.edu
A. G. Renner
Affiliation:
Department of Mathematics and Statistics, Utah State University, Logan, UT 84322, U.S.A. e-mail: [email protected]@cc.usu.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

A method, due to Élie Cartan, is used to give an algebraic classification of the non-reductive homogeneous pseudo-Riemannian manifolds of dimension four. Only one case with Lorentz signature can be Einstein without having constant curvature, and two cases with (2, 2) signature are Einstein of which one is Ricci-flat. 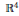If a four-dimensional non-reductive homogeneous pseudo-Riemannian manifold is simply connected, then it is shown to be diffeomorphic to ${{\mathbb{R}}^{4}}$. All metrics for the simply connected non-reductive Einstein spaces are given explicitly. There are no non-reductive pseudo-Riemannian homogeneous spaces of dimension two and none of dimension three with connected isotropy subgroup.

Keywords

53C30Homogeneous pseudo-RiemannianEinstein space
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 58 , Issue 2 , 01 April 2006 , pp. 282 - 311
Copyright
Copyright © Canadian Mathematical Society 2006

References

[1] Bérard-Bergery, L., Les espaces homogènes riemanniens de dimension 4. Textes Math. 3, CEDIC, Paris, 1981, pp. 40–60.Google Scholar
[2] Besse, A., Einstein Manifolds. Ergebnisse der Mathematik und ihrer Grenzgebiete 3, Springer-Verlag, Berlin 1987.Google Scholar
[3] Cartan, É., Leçons sur la géométrie des espaces de Riemann. Second edition. Gauthier-Villars, Paris, 1951.Google Scholar
[4] Chevalley, C., On the topological structure of solvable groups. Ann. of Math. 42(1941), 668–675.Google Scholar
[5] Gadea, P. M. and Oubiña, J. A., Reductive homogeneous pseudo-Riemannian manifolds. Monatsh. Math. 124(1997), no. 1, 17–34.Google Scholar
[6] Ghanam, R. and Thompson, G., The holonomy Lie algebras of neutral metrics in dimension four. J. Math. Phys. 42(2001), no. 5, 2266–2284.Google Scholar
[7] Ishihara, S., Homogeneous Riemannian spaces of four dimension. J. Math. Soc. Japan 7(1955), 345–370.Google Scholar
[8] Jensen, G., Geometry of homogeneous Einstein spaces of dimension four. J. Differential Geometry 3(1969), 309–349.Google Scholar
[9] Kobayashi, S. S. and Nomizu, K., Foundations of Differential Geometry. I. John Wiley, New York, 1996.Google Scholar
[10] Komrakov, B. Jr., Einstein-Maxwell equation on four-dimensional homogeneous spaces. Lobachevskii J. Math. 8(2001), 33–165 (electronic).Google Scholar
[11] Koornwinder, T. H. Invariant differential operators on nonreductive homogeneous spaces. Afdeling Zuivere Wiskunde 153, Mathematisch Centrum, Amsterdam, 1981, pp. 1–15; http://arXiv.org/abs/math/0008116. Google Scholar
[12] Kowalski, O. and Szenthe, J., On the existence of homogeneous geodesics in homogeneous Riemannian manifolds. Geom. Dedicata 81(2000), no. 1-3, pp. 209–214.Google Scholar
[13] Mostow, G. D. Factor spaces of solvable groups. Ann. of Math. 60(1954), 1–27.Google Scholar
[14] Onishchik, A. L. (Ed.), Lie Groups and Lie Algebras. I. Encyclopaedia Math. Sci. 20, Springer, Berlin, 1993.Google Scholar
[15] Patera, J., Winternitz, P., and Zassenhaus, H., Continuous subgroups of the fundamental groups of physics I. General method and the Poincaré group. J. Math. Phys. 16(1975), 1597–1614.Google Scholar
[16] Patera, J., Sharp, R. T. Winternitz, P., and Zassenhaus, H., Invariants of real low dimension Lie algebras. J. Mathematical Phys. 17(1976), no. 6, 986–994.Google Scholar
[17] Patera, J., Sharp, R. T. Winternitz, P., and Zassenhaus, H., Continuous subgroups of the fundamental groups of physics. III. The de-Sitter groups. J. Mathematical Phys. 18(1977), no. 12, 2259–2288.Google Scholar
[18] Petrov, A. Z., Einstein Spaces. Pergamon Press, Oxford, 1969.Google Scholar
[19] Wolf, J., Spaces of Constant Curvature. Fifth edition. Publish or Perish, Houston, TX, 1984.Google Scholar