Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Axvig, Nathan
Dreher, Deanna
Morrison, Katherine
Psota, Eric
Perez, Lance C.
and
Walker, Judy L.
2008.
Towards universal cover decoding.
p.
1.
Jorgensen, Palle E. T.
2009.
Analysis of unbounded operators and random motion.
Journal of Mathematical Physics,
Vol. 50,
Issue. 11,
Myasnikov, Alexei
and
Osin, Denis
2011.
Algorithmically finite groups.
Journal of Pure and Applied Algebra,
Vol. 215,
Issue. 11,
p.
2789.
Altisen, Karine
Devismes, Stéphane
Gerbaud, Antoine
and
Lafourcade, Pascal
2012.
Structural Information and Communication Complexity.
Vol. 7355,
Issue. ,
p.
254.
Stadlbauer, Manuel
2013.
An extension of Kesten’s criterion for amenability to topological Markov chains.
Advances in Mathematics,
Vol. 235,
Issue. ,
p.
450.
Fitzner, Robert
and
van der Hofstad, Remco
2013.
Non-backtracking Random Walk.
Journal of Statistical Physics,
Vol. 150,
Issue. 2,
p.
264.
Jaerisch, Johannes
2014.
Fractal models for normal subgroups of Schottky groups.
Transactions of the American Mathematical Society,
Vol. 366,
Issue. 10,
p.
5453.
Angel, Omer
Friedman, Joel
and
Hoory, Shlomo
2014.
The non-backtracking spectrum of the universal cover of a graph.
Transactions of the American Mathematical Society,
Vol. 367,
Issue. 6,
p.
4287.
Abért, Miklós
Glasner, Yair
and
Virág, Bálint
2014.
Kesten’s theorem for invariant random subgroups.
Duke Mathematical Journal,
Vol. 163,
Issue. 3,
Dougall, Rhiannon
and
Sharp, Richard
2016.
Amenability, critical exponents of subgroups and growth of closed geodesics.
Mathematische Annalen,
Vol. 365,
Issue. 3-4,
p.
1359.
Abért, Miklós
Glasner, Yair
and
Virág, Bálint
2016.
The measurable Kesten theorem.
The Annals of Probability,
Vol. 44,
Issue. 3,
Le Goff, Line C.
and
Raimond, Olivier
2018.
Vertex reinforced non-backtracking random walks: an example of path formation.
Electronic Journal of Probability,
Vol. 23,
Issue. none,
Anantharaman, Nalini
and
Sabri, Mostafa
2019.
Recent results of quantum ergodicity on graphs and further investigation.
Annales de la Faculté des sciences de Toulouse : Mathématiques,
Vol. 28,
Issue. 3,
p.
559.
Hermon, Jonathan
2019.
Reversibility of the non-backtracking random walk.
Annales de l'Institut Henri Poincaré, Probabilités et Statistiques,
Vol. 55,
Issue. 4,
Le Masson, Etienne
and
Sabri, Mostafa
2020.
$$L^p$$ Norms and Support of Eigenfunctions on Graphs.
Communications in Mathematical Physics,
Vol. 374,
Issue. 1,
p.
211.
Anantharaman, Nalini
Ingremeau, Maxime
Sabri, Mostafa
and
Winn, Brian
2021.
Absolutely Continuous Spectrum for Quantum Trees.
Communications in Mathematical Physics,
Vol. 383,
Issue. 1,
p.
537.
Nestoridi, Evita
and
Sarnak, Peter
2023.
Bounded Cutoff Window for the Non-backtracking Random Walk on Ramanujan Graphs.
Combinatorica,
Vol. 43,
Issue. 2,
p.
367.
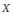 $X$ be a locally finite, connected graph without vertices of degree 1. Non-backtracking random walk moves at each step with equal probability to one of the “forward” neighbours of the actual state, i.e., it does not go back along the preceding edge to the preceding state. This is not a Markov chain, but can be turned into a Markov chain whose state space is the set of oriented edges of
$X$ be a locally finite, connected graph without vertices of degree 1. Non-backtracking random walk moves at each step with equal probability to one of the “forward” neighbours of the actual state, i.e., it does not go back along the preceding edge to the preceding state. This is not a Markov chain, but can be turned into a Markov chain whose state space is the set of oriented edges of 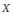 $X$. Thus we obtain for infinite
$X$. Thus we obtain for infinite 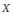 $X$ that the
$X$ that the  $n$-step non-backtracking transition probabilities tend to zero, and we can also compute their limit when
$n$-step non-backtracking transition probabilities tend to zero, and we can also compute their limit when 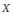 $X$ is finite. This provides a short proof of an old result concerning cogrowth of groups, and makes the extension of that result to arbitrary regular graphs rigorous. Even when
$X$ is finite. This provides a short proof of an old result concerning cogrowth of groups, and makes the extension of that result to arbitrary regular graphs rigorous. Even when 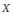 $X$ is non-regular, but small cycles are dense in
$X$ is non-regular, but small cycles are dense in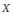 $X$, we show that the graph
$X$, we show that the graph 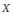 $X$ is non-amenable if and only if the non-backtracking
$X$ is non-amenable if and only if the non-backtracking  $n$-step transition probabilities decay exponentially fast. This is a partial generalization of the cogrowth criterion for regular graphs which comprises the original cogrowth criterion for finitely generated groups of Grigorchuk and Cohen.
$n$-step transition probabilities decay exponentially fast. This is a partial generalization of the cogrowth criterion for regular graphs which comprises the original cogrowth criterion for finitely generated groups of Grigorchuk and Cohen.

