Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-26T00:31:23.274Z Has data issue: false hasContentIssue false
  • English
  • Français

Necessary and Sufficient Conditions for Hypoellipticity for a Class of Convolution Operators

Published online by Cambridge University Press:  20 November 2018

Luo Xuebo
Luo Xuebo*
Affiliation:
Department of Mathematics Lanzhou University Lanzhou 730000 China
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper the Corwin's conjecture is proved, which says that if d is a function analytic near ∞, then the hypoellipticity of the convolution operator Ad, defined by 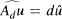for every u ∊ S'(ℝn), implies that P(x)/ logx → ∞ as x → ∞, where P(x) is the distance from x ∊ ℝn to the set of complex zeros of d.

Keywords

35H2522E27
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 46 , Issue 1 , 01 February 1994 , pp. 212 - 224
Copyright
Copyright © Canadian Mathematical Society 1994

Footnotes

Supported by NNFC and Special Fund for Doctoral Program in University.

References

1. Corwin, L., Necessary and Sufficient Conditions for Hypoellipticity for Certain Left Invariant Operators on Nilpotent Groups, Comm. PDE (9) 1(1984), 1–32.Google Scholar
2. Hormander, L., The Analysis of Linear Partial Differential Operators, If Springer-Verlag, Berlin, 1983.Google Scholar
3. Narasimhan, R., Several Complex Variables, The University of Chicago Press, Chicago and London, 1971.Google Scholar
4. Porter, M. H. and Weinberger, H. F., Maximum Principles in Differential Equations, Prentice-Hall, Englewood Cliffs, New Jersey, 1967.Google Scholar
5. Barros-Neto, J., An Introduction to the Theory of Distributions, Marcel Dekker, New York, 1973.Google Scholar