Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Çömez, Doğan
1998.
Moving Averages of Superadditive Processes with Respect to $L_p$-Contractions, $1\lt p\lt \infty$.
Rocky Mountain Journal of Mathematics,
Vol. 28,
Issue. 3,
Çömez, Doğan
and
Litvinov, Semyon
2000.
Norm Convergence of Moving Averages for $\tau$-Integrable Operators.
Rocky Mountain Journal of Mathematics,
Vol. 30,
Issue. 4,
Lee, George
2024.
Constructing the Oseledets decomposition with subspace growth estimates.
Transactions of the American Mathematical Society, Series B,
Vol. 11,
Issue. 11,
p.
396.


 be a semigroup of measure preserving transformations on a measure space (Ω, ℱ, μ). The main result of the paper is the proof of a.e. convergence for the moving averages
be a semigroup of measure preserving transformations on a measure space (Ω, ℱ, μ). The main result of the paper is the proof of a.e. convergence for the moving averages 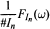
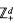 satisfying the "cone-condition". The identification of the limit is given. A moving local theorem is also proved.
satisfying the "cone-condition". The identification of the limit is given. A moving local theorem is also proved.