Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-26T00:51:27.572Z Has data issue: false hasContentIssue false
  • English
  • Français

Monocoreflective Subcategories in General Topology

Published online by Cambridge University Press:  20 November 2018

R. Grant Woods
R. Grant Woods*
Affiliation:
The University of Manitoba, Winnipeg, Manitoba
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 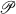be a full subcategory of a category is said to be coreflective in if for each object X in there exists an object X in 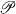and a morphism such that for each object P in 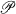and each morphism f : P ⟶ X there exists a unique morphism 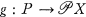such that .

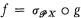Type
Research Article
Information
Canadian Journal of Mathematics , Volume 29 , Issue 6 , 01 December 1977 , pp. 1129 - 1140
Copyright
Copyright © Canadian Mathematical Society 1977

References

1. Bernstein, A. R., A new kind of compactness for topological spaces, Fund. Math. 66 (1969/70), 185–193.Google Scholar
2. Frohlich, O., Das Halbordnungssytem der topologischen Rdume auf einer Menge, Math. Ann. 156( 1964), 79–85.Google Scholar
3. Gillman, L. and Jerison, M., Rings of continuous functions (Van Nostrand Princeton, N.J. 1960).Google Scholar
4. Herrlich, H. and Strecker, G. E., Coreflective subcategories in general topology, Fund. Math. 73 (1971), 199–218.Google Scholar
5. Kennison, J. F., Reflective functions in general topology and elsewhere, Trans. Amer. Math. Soc. 118 (1965), 303–315.Google Scholar
6. Maclane, S., Categories for the working mathematician, G. T. M. 5 (Springer-Verlag, New York, 1971).Google Scholar
7. Porter, J. R. and Woods, R. G., Minimal extremally disconnected Hausdorff spaces, to appear, General Topology and its Applications.Google Scholar
8. Steiner, A. K., The lattice of topologies: structure and complementation, Trans. Amer. Math. Soc. 122 (1966), 379–398.Google Scholar
9. Walker, R. C., The Stone-Cech compactification, Band 83 Ergebnisse der Math, und ihrer Grenz. (Springer-Verlag, New York, 1974).Google Scholar
10. Woods, R. G., Topological extension properties, Trans. Amer. Math. Soc. 210 (1975), 365–386.Google Scholar