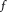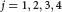Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Heittokangas, Janne
Ishizaki, Katsuya
Laine, Ilpo
and
Tohge, Kazuya
2021.
Exponential polynomials in the oscillation theory.
Journal of Differential Equations,
Vol. 272,
Issue. ,
p.
911.