Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-24T00:10:45.546Z Has data issue: false hasContentIssue false
  • English
  • Français

Mahler Measures as Linear Combinations of L–values of Multiple Modular Forms

Published online by Cambridge University Press:  20 November 2018

Detchat Samart
Detchat Samart*
Affiliation:
Department of Mathematics, Texas A&M University, College Station, TX 77843, USA. e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We study the Mahler measures of certain families of Laurent polynomials in two and three variables. Each of the known Mahler measure formulas for these families involves 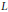$L$–values of at most one newform and/or at most one quadratic character. In this paper we show, either rigorously or numerically, that the Mahler measures of some polynomials are related to 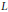$L$–values of multiple newforms and quadratic characters simultaneously. The results suggest that the number of modular 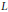$L$–values appearing in the formulas significantly depends on the shape of the algebraic value of the parameter chosen for each polynomial. As a consequence, we also obtain new formulas relating special values of hypergeometric series evaluated at algebraic numbers to special values of 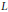$L$–functions.

Keywords

11F6733C20Mahler measuresEisenstein–Kronecker seriesL–functionshypergeometric series
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 67 , Issue 2 , April 2015 , pp. 424 - 449
Copyright
Copyright © Canadian Mathematical Society 2015

References

[1] Ahlgren, S., Ono, K., and Penniston, D., Zeta function of an infinite family of K3 surfaces. Amer. J. Math. 124(2002), 353–368. http://dx.doi.org/10.1353/ajm.2002.0007 Google Scholar
[2] Berndt, B. C., Ramanujan's notebooks. Part III. Springer–Verlag, New York, NY, 1991.Google Scholar
[3] Berndt, B. C., Bhargava, S., and Garvan, F. G., Ramanujan's theories of elliptic functions to alternative bases. Trans. Amer. Math. Soc. 347(1995), no. 11, 4163–4244.Google Scholar
[4] Bertin, M. J., Mesure de Mahler d’hypersurfaces K3. J. Number Theory 128(2008), no. 11, 2890–2913. http://dx.doi.org/10.1016/j.jnt.2007.12.012 Google Scholar
[5] Boyd, D.W., Mahler's measure and special values of L–functions. Experiment. Math. 7(1998), no. 1, 37–82. http://dx.doi.org/10.1080/10586458.1998.10504357 Google Scholar
[6] Chen, I. and Yui, N., Singular values of Thompson series. In: Groups, difference sets, and the Monster(Columbus, OH, 1993), Ohio State Univ. Math. Res. Inst. Publ., 4, de Gruyter, Berlin, 1996, pp. 255–326.Google Scholar
[7] Deninger, C., Deligne periods of mixed motives, K–theory and the entropy of certain Zn–actions. J. Amer. Math. Soc. 10(1997), no. 2, 259–281. http://dx.doi.org/10.1090/S0894-0347-97-00228-2 Google Scholar
[8] Glasser, M. L. and Zucker, I. J., Lattice sums. In: Perspectives in theoretical chemistry: Advances and perspectives, 5, Academic Press, New York, NY, 1980, pp. 67–139.Google Scholar
[9] Guillera, J. and Rogers, M., Mahler measure and the WZ algorithm. Proc. Amer. Math. Soc., to appear.Google Scholar
[10] Hartmann, H., Period– and mirror–maps for the quartic K3. Manuscripta Math. 141 2013, no. 3–4, 391–422. http://dx.doi.org/10.1007/s00229-012-0577-7Google Scholar
[11] Kurokawa, N. and Ochiai, H., Mahler measures via the crystalization. Comment. Math. Univ. St. Pauli 54(2005), no. 2, 121–137.Google Scholar
[12] Laĺn, M. N. and Rogers, M. D., Functional equations for Mahler measures of genus–one curves. Algebra Number Theory 1(2007), no. 1, 87–117. http://dx.doi.org/10.2140/ant.2007.1.87 Google Scholar
[13] Livné, R., otivic orthogonal two–dimensional representations of . Israel J. Math. 92(1995), no. 1–3, 149–156. http://dx.doi.org/10.1007/BF02762074 Google Scholar
[14] Long, L., Modularity of elliptic surfaces. Ph.D. Thesis, The Pennsylvania State University, Proquest LLC, Ann Arbor, MI, 2002.Google Scholar
[15] Long, L., On a Shioda–Inose structure of a family of K3surfaces. In: Calabi–Yau varieties and mirror symmetry, Fields Institute Communications, 38, American Mathematical Society, Providence, RI, 2003, pp. 201–207.Google Scholar
[16] Long, L., On Shioda–Inose structure of one–parameter families of K3surfaces. J. Number Theory, 109(2004), no. 2, 299–318.http://dx.doi.org/10.1016/j.jnt.2004.06.009 Google Scholar
[17] Morrison, D. R., On K3surfaces with large Picard number. Invent. Math. 75(1984), no. 1, 105–121. http://dx.doi.org/10.1007/BF01403093 Google Scholar
[18] Ono, K., The web of modularity: arithmetic of the coefficients of modular forms and q–series. BMS Regional Conference Series in Mathematics, 102, Conference Board of the Mathematical Sciences, Washington, DC; Amererican Mathematical Society, Providence, RI, 2004.Google Scholar
[19] Rodriguez Villegas, F., Modular Mahler measures. I. In: Topics in Number Theory (University Park, PA, 1997), Math. Appl., 467, Kluwer, Dordrecht, 1999, pp. 17–48.Google Scholar
[20] Rogers, M. D., Hypergeometric formulas for lattice sums and Mahler measures. Int. Math. Res. Not. IMRN 2011, no. 17, 4027–4058.Google Scholar
[21] Rogers, M. D., New 5F4hypergeometric transformations, three-variable Mahler measures, and formulas for 1/π Ramanujan J. 18(2009), no. 3, 327–340. http://dx.doi.org/10.1007/s11139-007-9040-x Google Scholar
[22] Rogers, M. and Yuttanan, B., Modular equations and lattice sums. In: Computational and Analytical Mathematics, Springer Proceedings in Mathematics, to appear.Google Scholar
[23] Rogers, M. and Zudilin, W., From L–series of elliptic curves to Mahler measures. Compos. Math. 148(2012), no. 2, 385–414. http://dx.doi.org/10.1112/S0010437X11007342 Google Scholar
[24] Rogers, M. and Zudilin, W., On the Mahler measure of + X + 1=X + Y + 1= Y. Int. Math. Res. Notices to appear.Google Scholar
[25] Samart, D., Three–variable Mahler measures and special values of modular and Dirichlet L–series. Ramanujan J. 32(2013) no. 2, 245–268. http://dx.doi.org/10.1007/s11139-013-9464-4 Google Scholar
[26] Samart, D., The elliptic trilogarithm and Mahler measures of K3surfaces. http://arxiv:1309.7730 Google Scholar
[27] Schütt, M., CM newforms with rational coefficients. Ramanujan J. 19(2009), no. 2, 187–205. http://dx.doi.org/10.1007/s11139-008-9147-8 Google Scholar
[28] Schütt, M., Two lectures on the arithmetic of K3 surfaces. In: Arithmetic and Geometry of K3 Surfaces and Calabi–Yau Threefolds, Fields Institute Communications, 67, Springer, New York, 2013, pp. 71–99.Google Scholar
[29] Schütt, M. and Shioda, T., Elliptic surfaces. In: Algebraic geometry in East Asia–Seoul 2008, Adv. Stud. Pure Math., 60, Math. Soc. Japan, Tokyo, 2010, pp. 51–160.Google Scholar
[30] Silverman, J. H., Advanced topics in the arithmetic of elliptic curves. Graduate Texts in Mathematics, 151, Springer–Verlag, New York, 1994.Google Scholar
[31] Weber, H., Lehrbuch der Algebra. Bd. III, F. Vieweg & Sohn, Braunschweig, 1908.Google Scholar
[32] Yui, N., Update on the moudularity of Calabi-Yau varieties. In: Calabi–Yau varieties and mirror symmetry, Fields Institute Communications, 38, American Mathematical Society, Providence RI, 2003, pp. 307–362.Google Scholar
[33] Yui, N. and Zagier, D., On the singular values of Weber modular functions. Math. Comp. 66(1997), no. 220, 1645–1662. http://dx.doi.org/10.1090/S0025-5718-97-00854-5 Google Scholar
[34] Zudilin, W., Regulator of modular units and Mahler measures. Math. Proc. Cambridge Philos. Soc. 156(2014), no. 2, 313–326.http://dx.doi.org/10.1017/S0305004113000765 Google Scholar