Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-26T00:45:22.406Z Has data issue: false hasContentIssue false
  • English
  • Français

A Local Ergodic Theorem on Lp

Published online by Cambridge University Press:  20 November 2018

J. R. Baxter  and
R. V. Chacon
J. R. Baxter
Affiliation:
University of British Columbia, Vancouver, British Columbia
R. V. Chacon
Affiliation:
University of British Columbia, Vancouver, British Columbia
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Two general types of pointwise ergodic theorems have been studied: those as t approaches infinity, and those as t approaches zero. This paper deals with the latter case, which is referred to as the local case.

Let (X, , μ) be a complete, σ-finite measure space. Let {Tt} be a strongly continuous one-parameter semi-group of contractions on 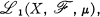, defined for t ≧ 0. For Tt positive, it was shown independently in [2] and [5] that

1.1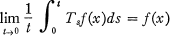

almost everywhere on X, for any f ∊ L1. The same result was obtained in [1], with the continuity assumption weakened to having it hold for t > 0.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 26 , Issue 5 , October 1974 , pp. 1206 - 1216
Copyright
Copyright © Canadian Mathematical Society 1974

References

1. Akcoglu, M. and Chacon, R., A local ratio theorem, Can. J. Math. 22 (1970), 545–552.Google Scholar
2. Krengel, U., A local ergodic theorem, Invent. Math. 6 (1969), 329–333.Google Scholar
3. Kubokawa, Y., Ergodic theorems for contraction semi-group (to appear).Google Scholar
4. Kubokawa, Y., local ergodic theorem for semi-group on (to appear).Google Scholar
5. Ornstein, D., The sum of the iterates of a positive operator, Advances in Probability and Related Topics (Edited by P. Ney) 2 (1970), 87–115.Google Scholar